Linear Inequalities Class 11 Maths NCERT Solutions are extremely helpful while doing your homework. NCERT Solutions for Class 11 Maths Chapter 6 Linear Inequalities All Exercises were prepared by Experienced LearnCBSE.in Teachers.

Free download NCERT Solutions for Class 11 Maths Chapter 6 Linear Inequalities Ex 6.1, Ex 6.2, Ex 6.3 and Miscellaneous Exercise PDF in Hindi Medium as well as in English Medium for CBSE, Uttarakhand, Bihar, MP Board, Gujarat Board, BIE, Intermediate and UP Board students, who are using NCERT Books based on updated CBSE Syllabus for the session 2019-20.

NCERT Solutions for Class 11 Maths Chapter 6 Linear Inequalities

Topics and Sub Topics in Class 11 Maths Chapter 6 Linear Inequalities:

Section Name	Topic Name
6	Linear Inequalities
6.1	Introduction
6.2	Inequalities
6.3	Algebraic Solutions of Linear Inequalities in One Variable and their Graphical Representation
6.4	Graphical Solution of Linear Inequalities in Two Variables
6.5	Solution of System of Linear Inequalities in Two Variables

NCERT Solutions for Class 11 Maths Chapter 6 Exercise 6.1

Question 1:

Ans:

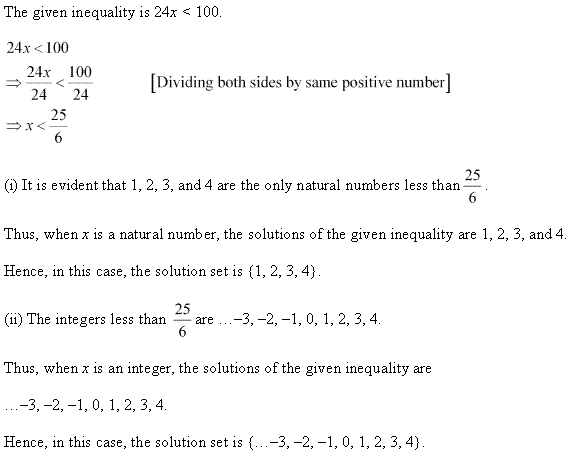
Question 2:

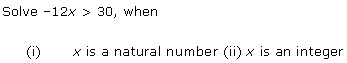
Ans:

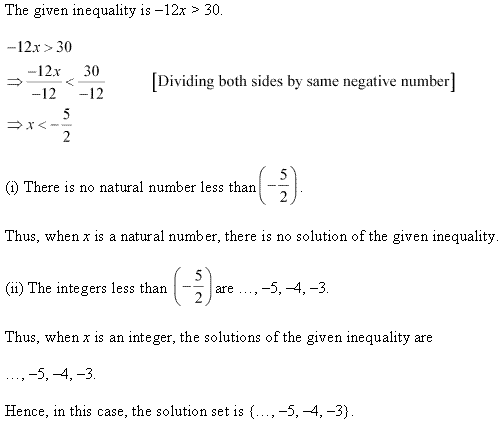
Question 3:

Ans:

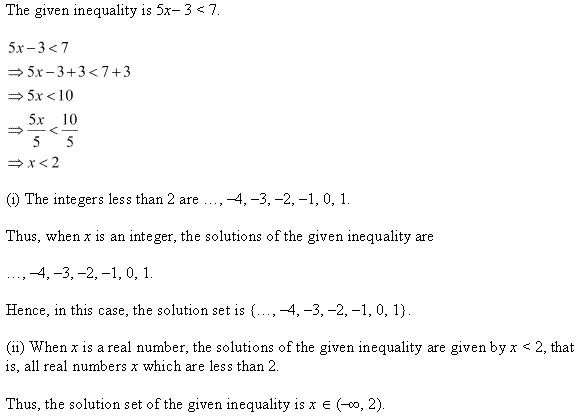
Question 4:

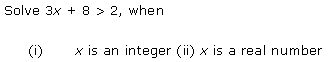
Ans:

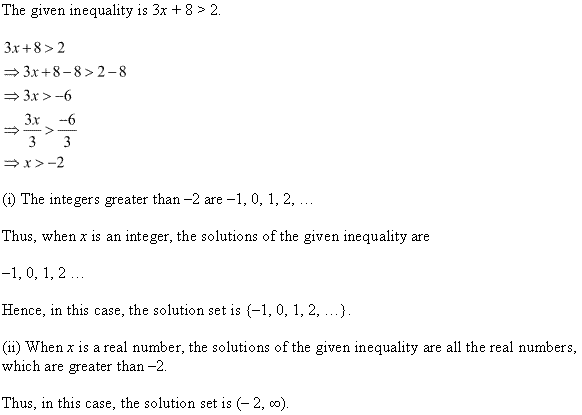
Question 5:

Ans:

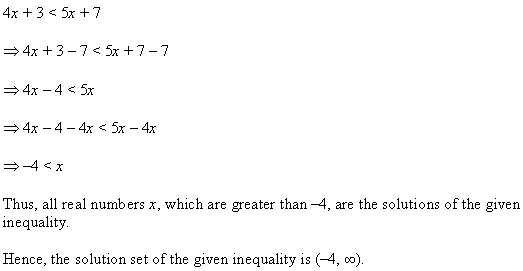
Question 6:

Ans:

Question 7:

Ans:

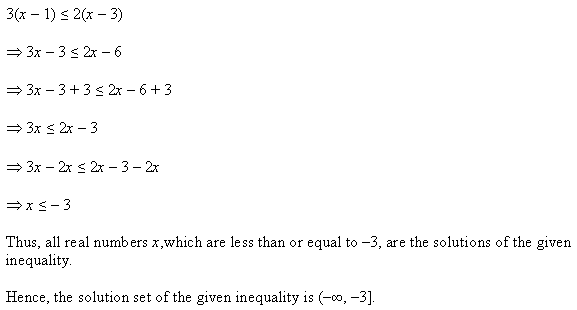
Question 8:

Ans:

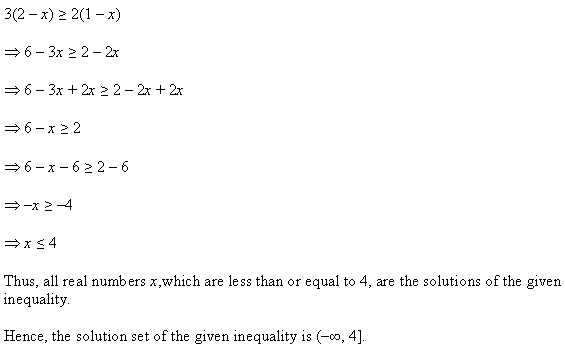
Question 9:

Ans:

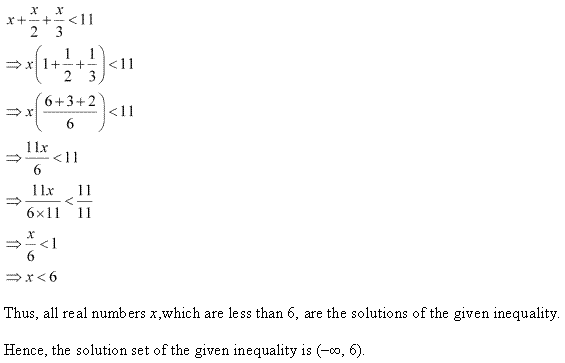
Question 10:

Ans:

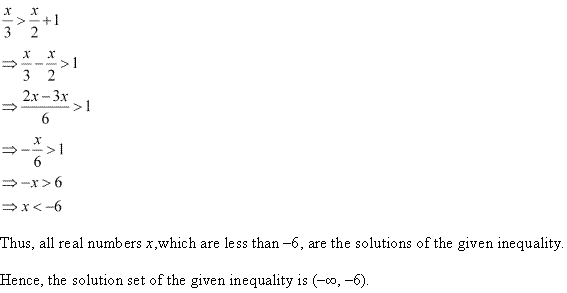
Question 11:

Ans:

Question 12:

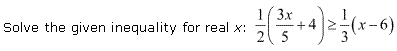
Ans:

Question 13:

Ans:

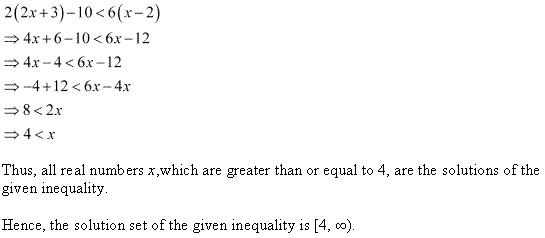
Question 14:

Ans:

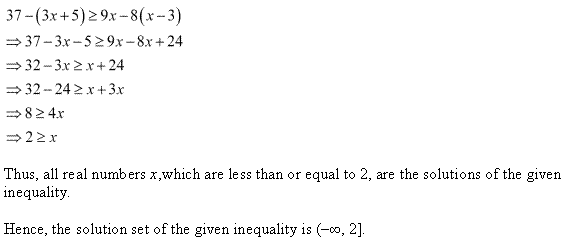
Question 15:

Ans:

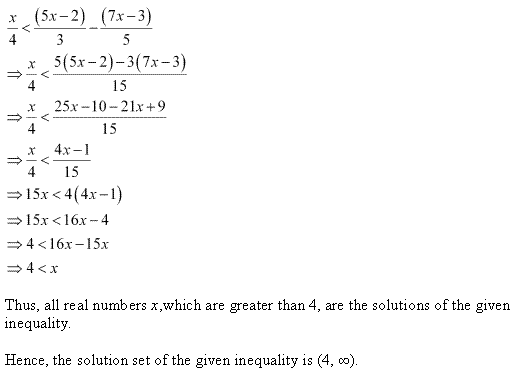
Question 16:

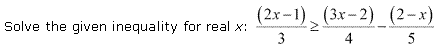
Ans:

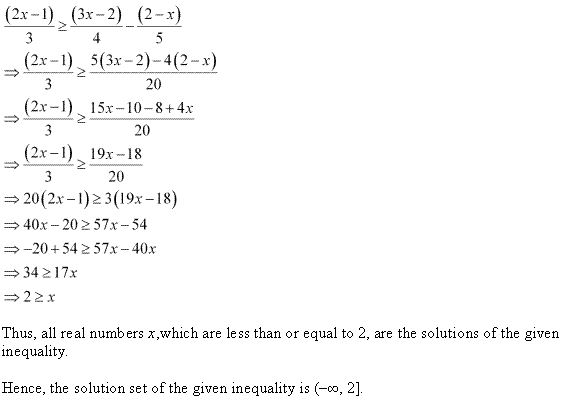
Question 17:

Ans:

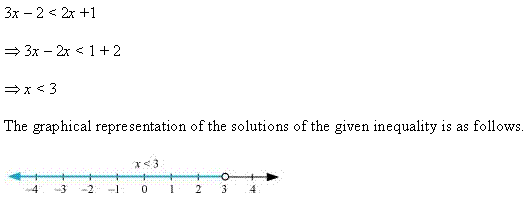
Question 18:

Ans:

Question 19:

Ans:

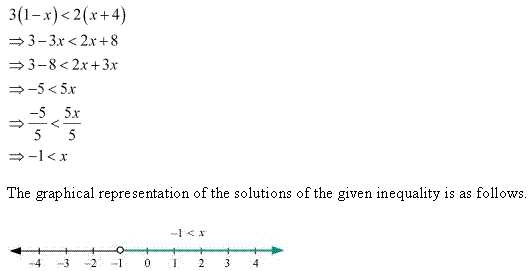
Question 20:

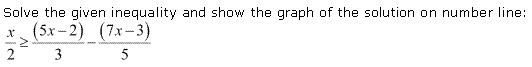
Ans:

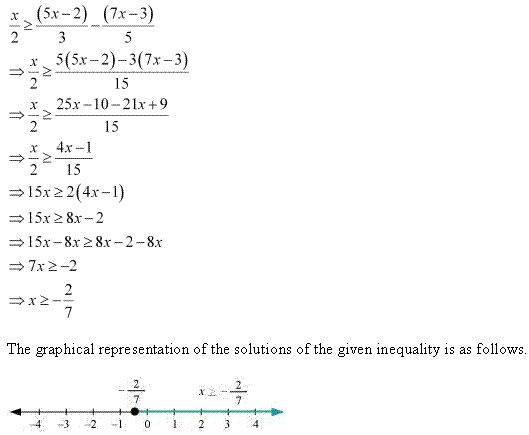
Question 21:

Ans:

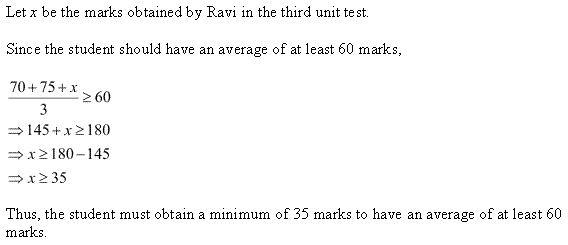
Question 22:

Ans:

Question 23:

Ans:

Question 24:

Ans:

Question 25:

Ans:

Question 26:

Ans:

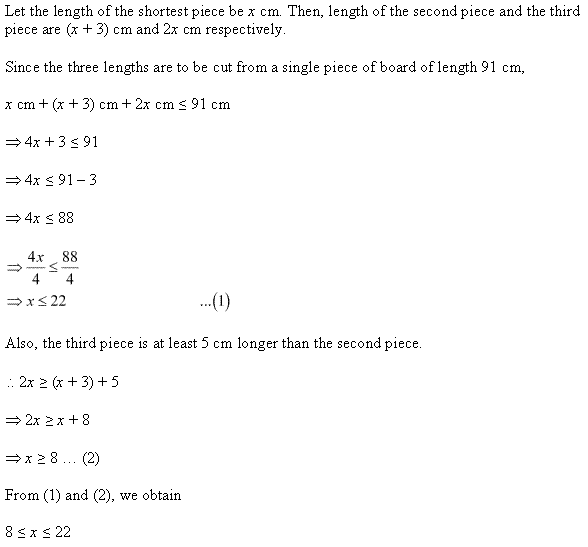
NCERT Solutions for Class 11 Maths Chapter 6 Linear Inequalities (रैखिक असमिकाएँ) Hindi medium Ex 6.1

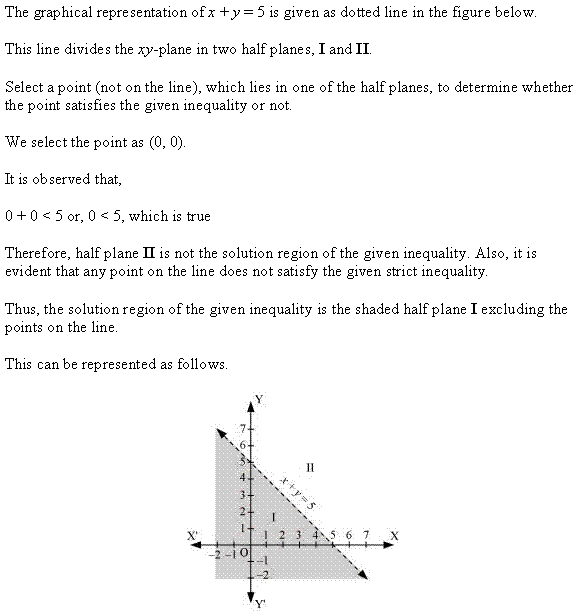
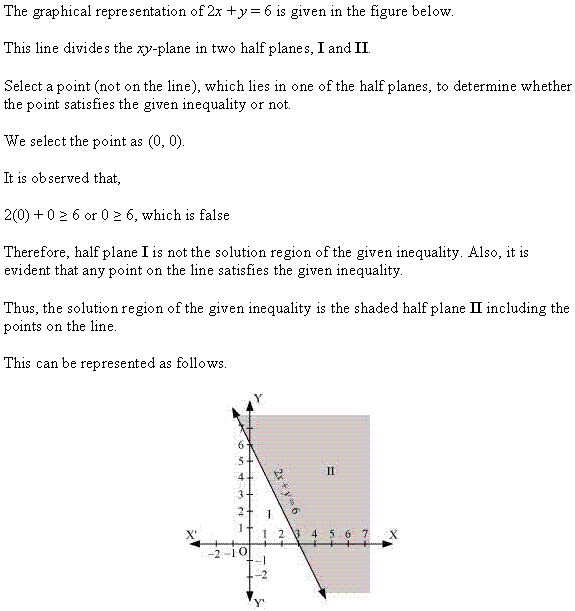
NCERT Solutions for Class 11 Maths Chapter 6 Exercise 6.2

Ex 6.2 Class 11 Maths Question 1:

Ans:

Ex 6.2 Class 11 Maths Question 2:

Ans:

Ex 6.2 Class 11 Maths Question 3:

Ans:

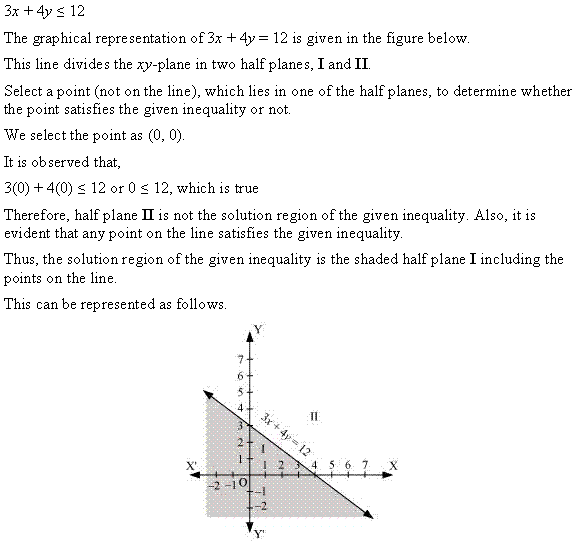
Ex 6.2 Class 11 Maths Question 4:

Ans:

Ex 6.2 Class 11 Maths Question 5:

Ans:

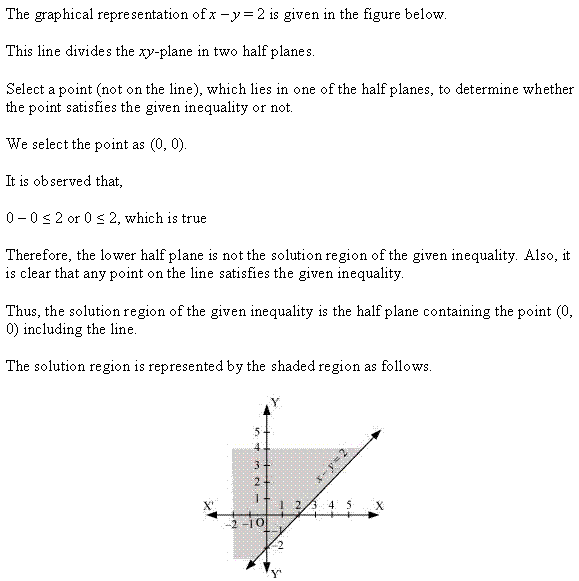
Ex 6.2 Class 11 Maths Question 6:

Ans:

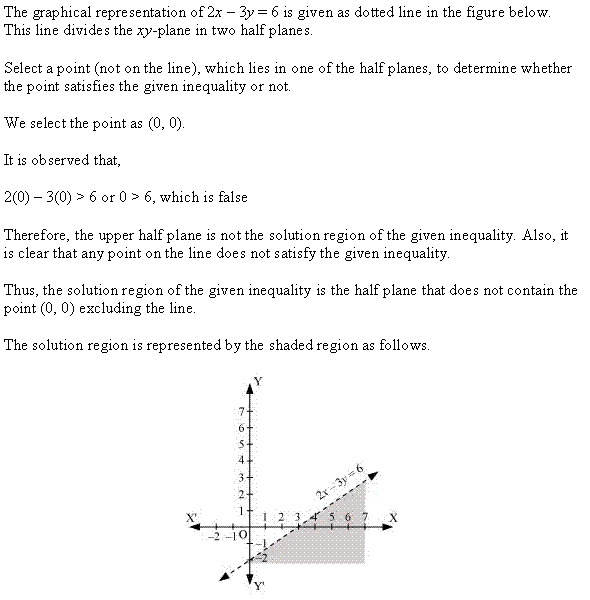
Ex 6.2 Class 11 Maths Question 7:

Ans:

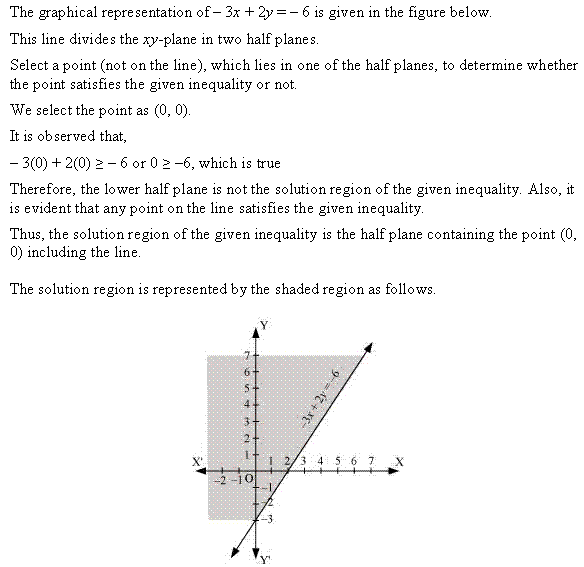
Ex 6.2 Class 11 Maths Question 8:

Ans:

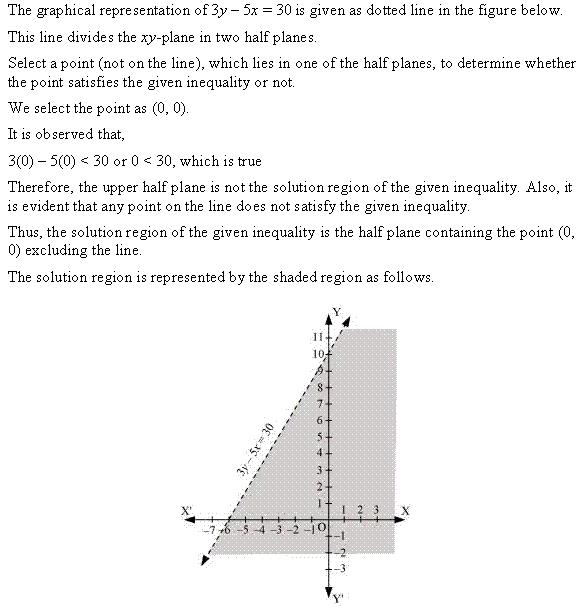
Ex 6.2 Class 11 Maths Question 9:

Ans:

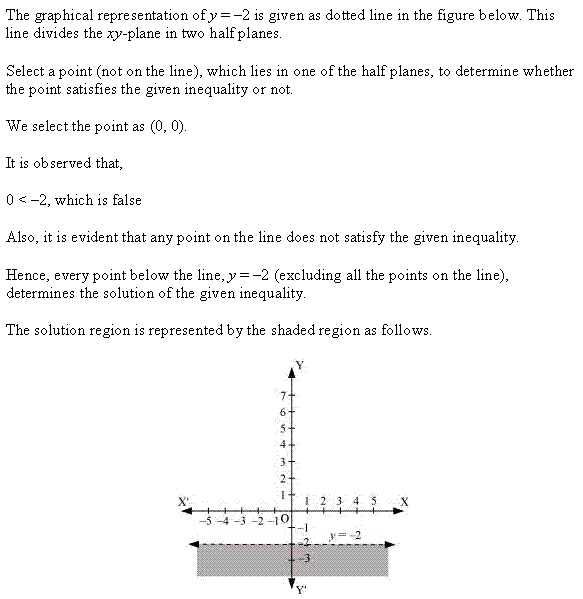
Ex 6.2 Class 11 Maths Question 10:

Ans:

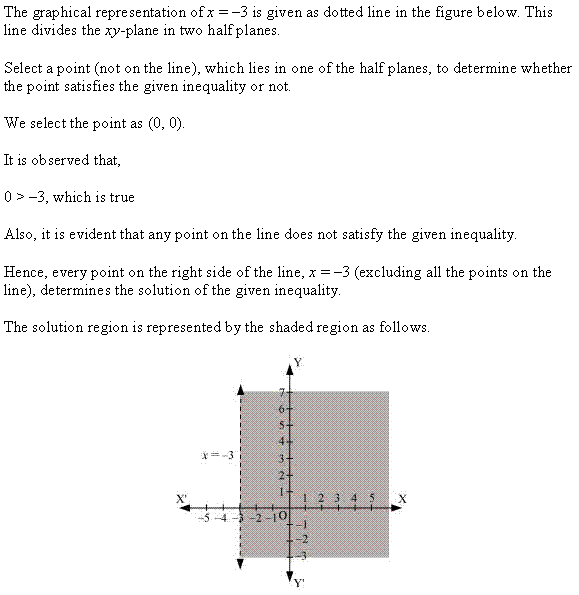
NCERT Solutions for Class 11 Maths Chapter 6 Exercise 6.3

Ex 6.3 Class 11 Maths Question 1:

Ans:

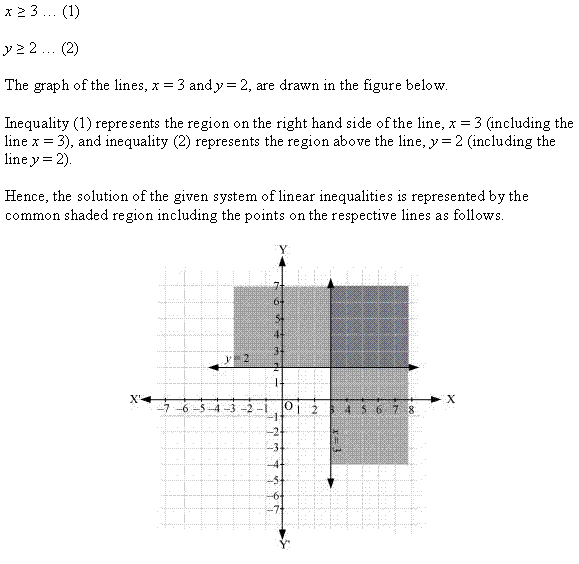
Ex 6.3 Class 11 Maths Question 2:

Ans:

Ex 6.3 Class 11 Maths Question 3:

Ans:

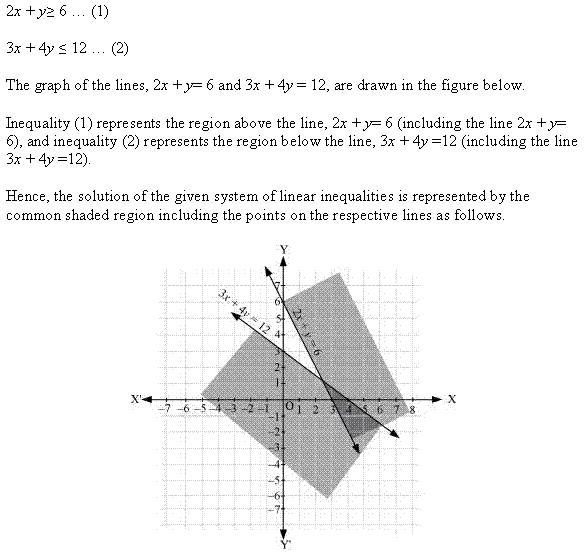
Ex 6.3 Class 11 Maths Question 4:

Ans:

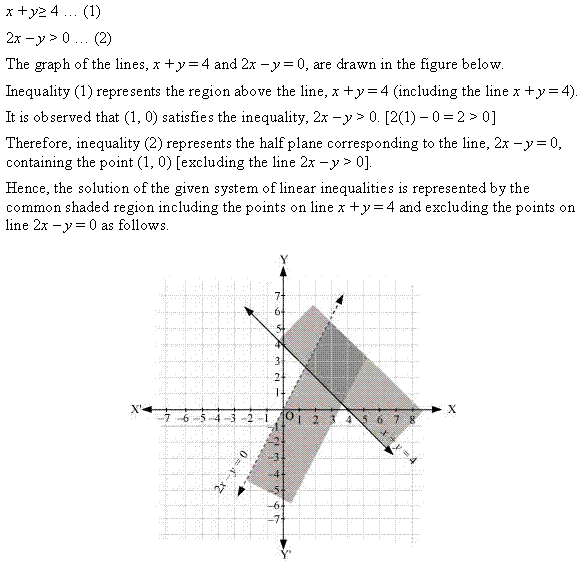
Ex 6.3 Class 11 Maths Question 5:

Ans:

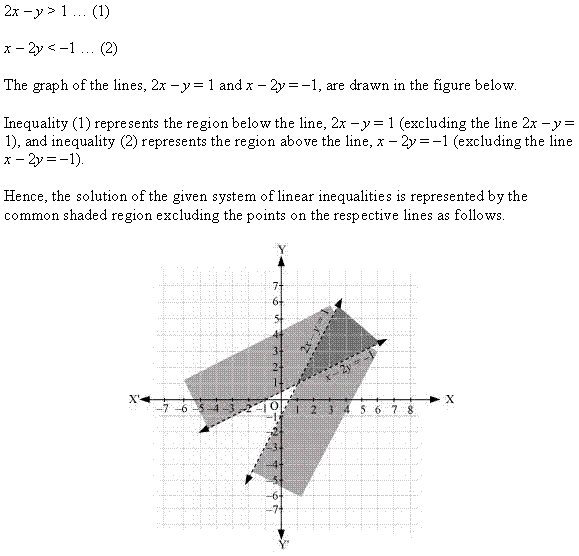
Ex 6.3 Class 11 Maths Question 6:

Ans:

Ex 6.3 Class 11 Maths Question 7:

Ans:

Ex 6.3 Class 11 Maths Question 8:

Ans:

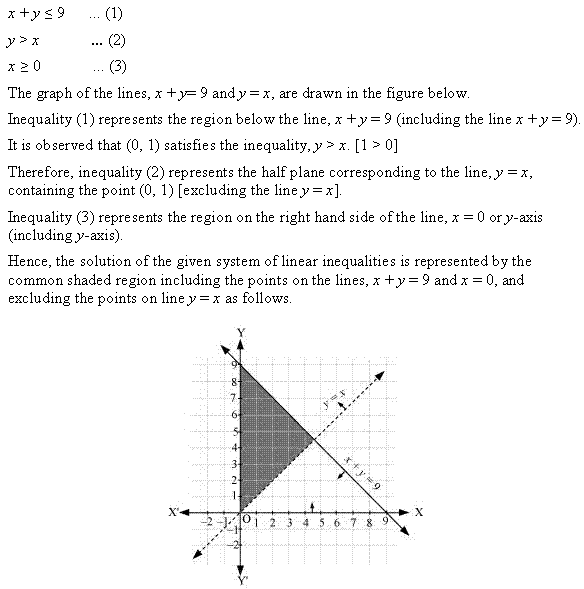
Ex 6.3 Class 11 Maths Question 9:

Ans:

Ex 6.3 Class 11 Maths Question 10:

Ans:

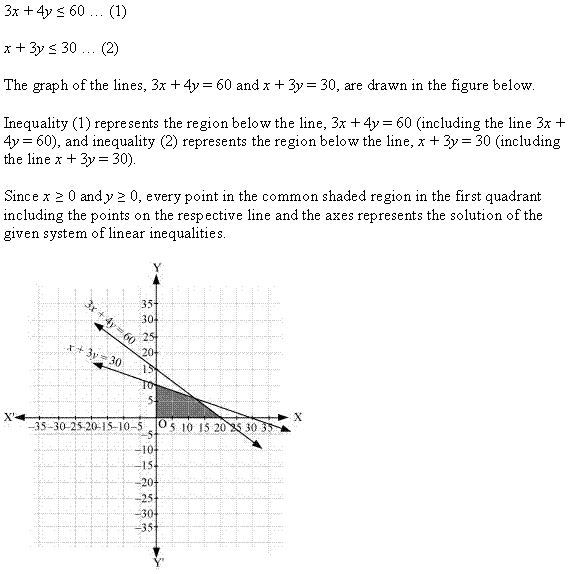
Ex 6.3 Class 11 Maths Question 11:

Ans:

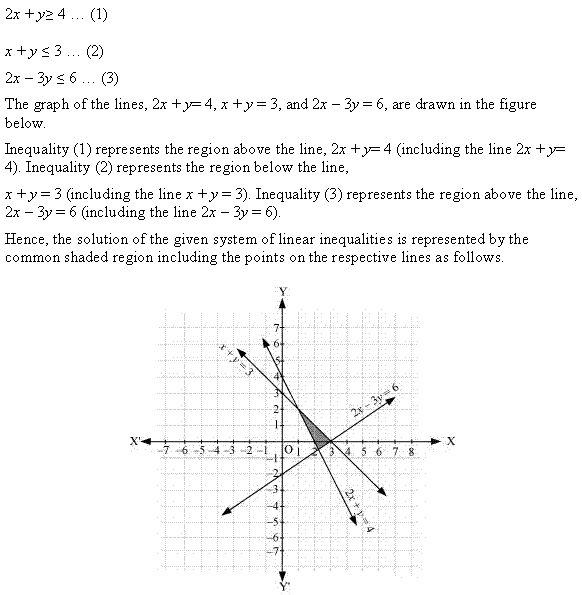
Ex 6.3 Class 11 Maths Question 12:

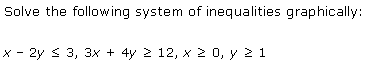
Ans:

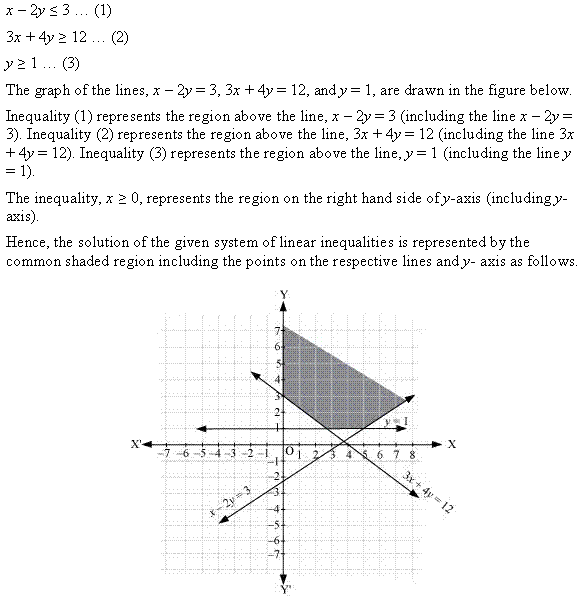
Ex 6.3 Class 11 Maths Question 13:

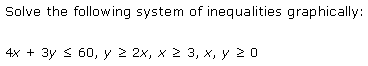
Ans:

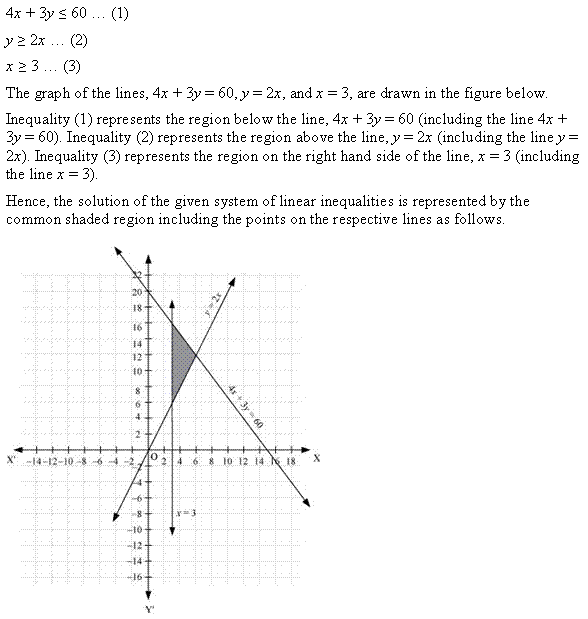
Ex 6.3 Class 11 Maths Question 14:

Ans:

Ex 6.3 Class 11 Maths Question 15:

Ans:

NCERT Solutions for Class 11 Maths Chapter 6 Miscellaneous Solutions

Miscellaneous Exercise Class 11 Maths Question 1:

Ans:

Miscellaneous Exercise Class 11 Maths Question 2:

Ans:

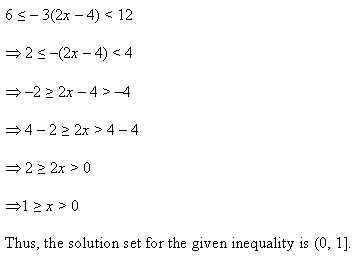
Miscellaneous Exercise Class 11 Maths Question 3:

Ans:

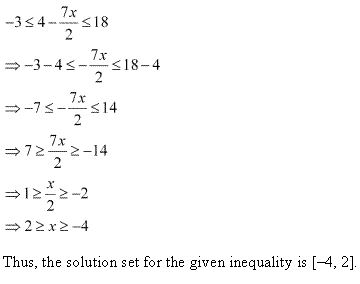
Miscellaneous Exercise Class 11 Maths Question 4:

Ans:

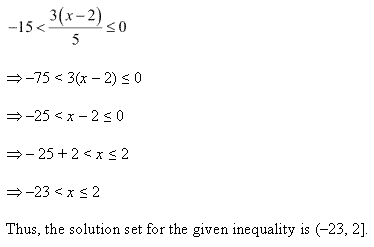
Miscellaneous Exercise Class 11 Maths Question 5:

Ans:

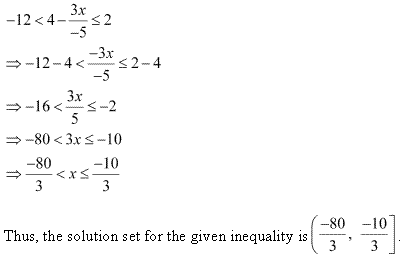
Miscellaneous Exercise Class 11 Maths Question 6:

Ans:

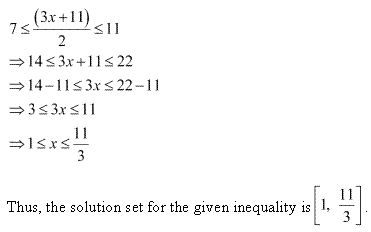
Miscellaneous Exercise Class 11 Maths Question 7:

Ans:

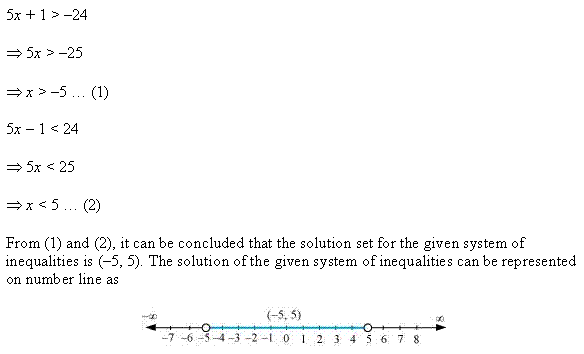
Miscellaneous Exercise Class 11 Maths Question 8:

Ans:

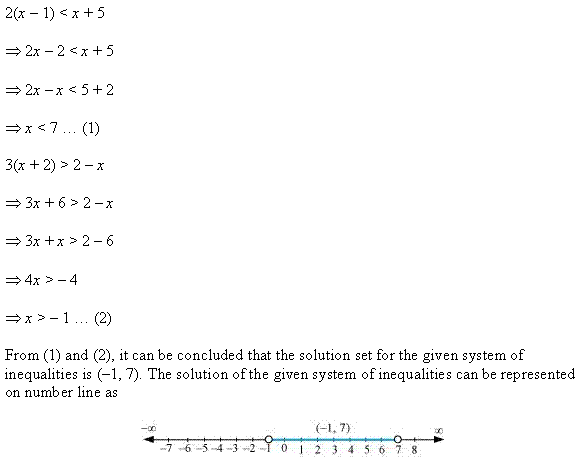
Miscellaneous Exercise Class 11 Maths Question 9:

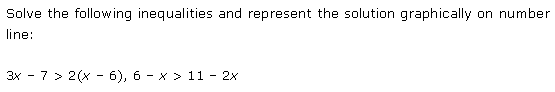
Ans:

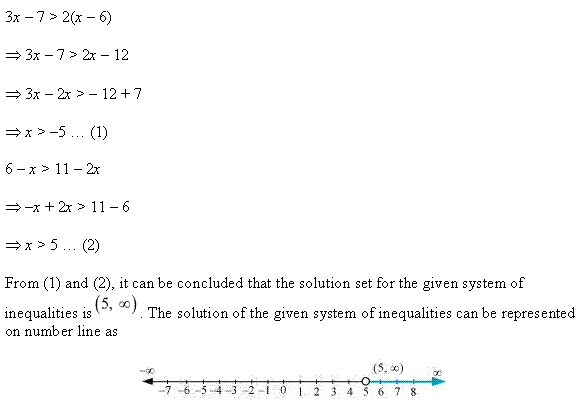
Miscellaneous Exercise Class 11 Maths Question 10:

Ans:

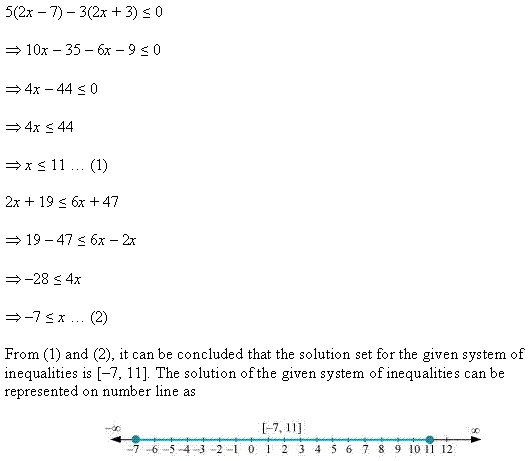
Miscellaneous Exercise Class 11 Maths Question 11:

Ans:

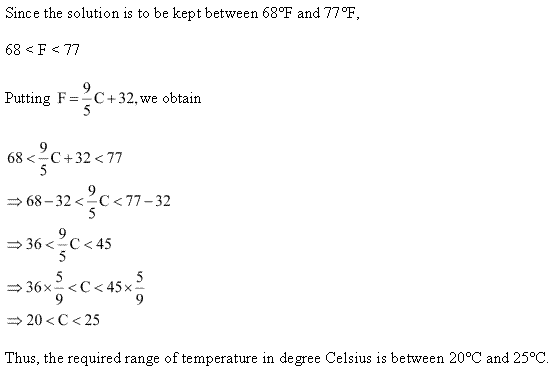
Miscellaneous Exercise Class 11 Maths Question 12:

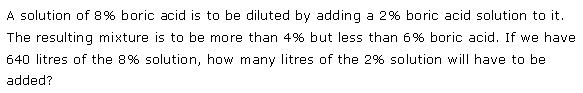
Ans:

Miscellaneous Exercise Class 11 Maths Question 13:

Ans:

Miscellaneous Exercise Class 11 Maths Question 14:

Ans:

NCERT Solutions for Class 11 Maths Chapter 6 .1 Linear Inequalities Ex 6 in Hindi

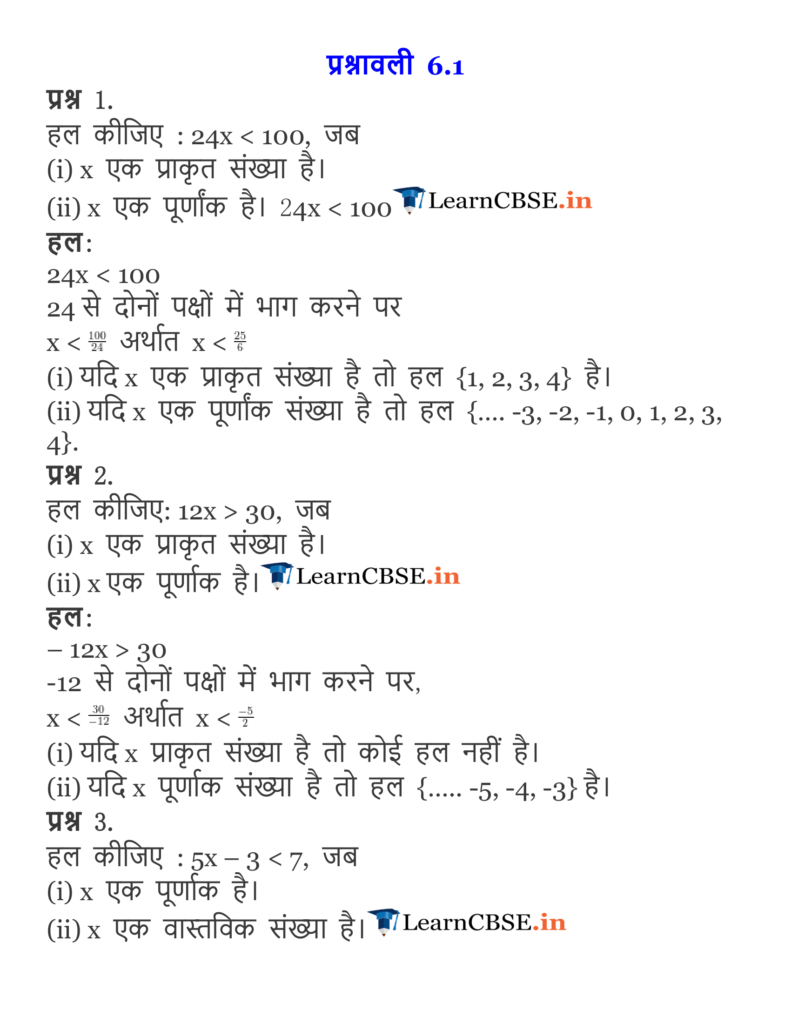
Ex 6.1 Class 11 Maths प्रश्न 1.
हल कीजिए : 24x < 100, जब
(i) x एक प्राकृत संख्या है।
(ii) x एक पूर्णांक है। 24x < 100
हल:
24x < 100
24 से दोनों पक्षों में भाग करने पर
x < 10024 अर्थात x < 256
(i) यदि x एक प्राकृत संख्या है तो हल {1, 2, 3, 4} है।
(ii) यदि x एक पूर्णांक संख्या है तो हल {…. -3, -2, -1, 0, 1, 2, 3, 4}.

Ex 6.1 Class 11 Maths प्रश्न 2.
हल कीजिए: 12x > 30, जब
(i) x एक प्राकृत संख्या है।
(ii) x एक पूर्णाक है।
हल:
– 12x > 30
-12 से दोनों पक्षों में भाग करने पर,
x < 30−12 अर्थात x < −52
(i) यदि x प्राकृत संख्या है तो कोई हल नहीं है।
(ii) यदि x पूर्णाक संख्या है तो हल {….. -5, -4, -3} है।

Ex 6.1 Class 11 Maths प्रश्न 3.
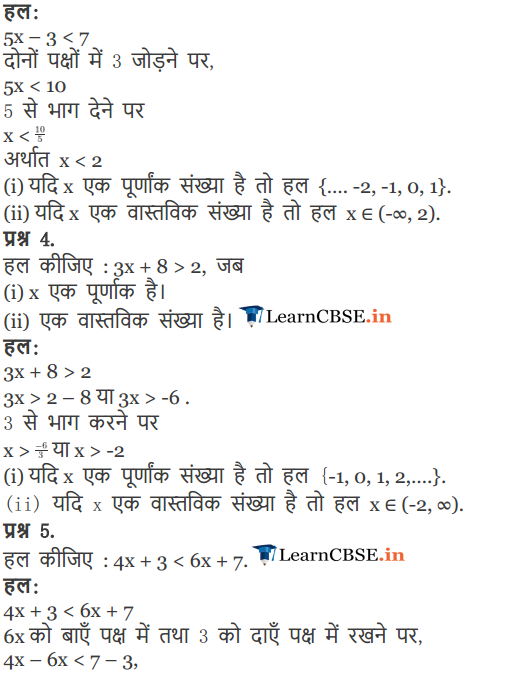
हल कीजिए : 5x – 3 < 7, जब
(i) x एक पूर्णाक है।
(ii) x एक वास्तविक संख्या है।
हल:
5x – 3 < 7
दोनों पक्षों में 3 जोड़ने पर,
5x < 10
5 से भाग देने पर
x < 105
अर्थात x < 2
(i) यदि x एक पूर्णांक संख्या है तो हल {…. -2, -1, 0, 1}.
(ii) यदि x एक वास्तविक संख्या है तो हल x ∈ (-∞, 2).

Ex 6.1 Class 11 Maths प्रश्न 4.
हल कीजिए : 3x + 8 > 2, जब
(i) x एक पूर्णाक है।
(ii) एक वास्तविक संख्या है।
हल:
3x + 8 > 2
3x > 2 – 8 या 3x > -6 .
3 से भाग करने पर
x > −63 या x > -2
(i) यदि x एक पूर्णांक संख्या है तो हल {-1, 0, 1, 2,….}.
(ii) यदि x एक वास्तविक संख्या है तो हल x ∈ (-2, ∞).

Ex 6.1 Class 11 Maths प्रश्न 5.
हल कीजिए : 4x + 3 < 6x + 7.
हल:
4x + 3 < 6x + 7
6x को बाएँ पक्ष में तथा 3 को दाएँ पक्ष में रखने पर,
4x – 6x < 7 – 3,
-2x < 4 -2 से भाग देने पर, x > 4−2 या x > -2
दी हुई असमिका का हल है: x = (-2, ∞).

Ex 6.1 Class 11 Maths प्रश्न 6.
हल कीजिए : 3x – 7 > 5x – 1
हल:
3x -7 > 5x – 1
5x को बाएँ पक्ष में और 7 को दाएँ पक्ष में रखने पर,
3x – 5x > -1 + 7
या
-2x > 6
-2x से भाग देने पर।
x < -3
दी हुई असमिका का हल है x ∈ (-∞, – 3).

Ex 6.1 Class 11 Maths प्रश्न 7.
हल कीजिए : 3(x – 1) ≤ 2 (x – 3).
हल:
असमिका
3(x – 1) ≤ 2 (x – 3)
3x – 3 ≤ 2x – 6
2x को बाएँ पक्ष में और 3 को दाएँ पक्ष में रखने पर,
3x – 2 ≤ 3 – 6
x < – 3
हल है : x ∈ (-∞, – 3].

Ex 6.1 Class 11 Maths प्रश्न 8.
हल कीजिए : 3 (2 – x) ≥ 2 (1 – x).
हल:
दी हुई असमिका 3(2 – x) ≥ 2 (1 – x)
6 – 3x ≥ 2 – 2x
2x को बायीं ओर तथा 6 को दायीं ओर रखने पर,
2x – 3x ≥ 2 – 6
या
-x ≥ -4 या x ≤ 4
हल है : x ∈ (-∞, 4]

Ex 6.1 Class 11 Maths प्रश्न 13.
हल कीजिए : 2 (2x + 3) – 10 < 6 (x – 2)
हल:
दी हुई असमिका 2 (2x + 3) – 10 < 6 (x – 2)
4x + 6 – 10 < 6x – 12
6x को बायीं ओर तथा -4 को दार्थी ओर रखने पर,
4 – 6x < -12 + 4
-2x < -8 (-1) से गुणा करने पर, x > 4
हल है :
x ∈ (4, ∞)

Ex 6.1 Class 11 Maths प्रश्न 14.
हल कीजिए : 37 – (3x + 5) ≥ 9x – 8(x – 3).
हल:
दी हुई असमिका 37 – (3x + 5) ≥ 9x – 8(x – 3)
37 – 3x – 5 ≥ 9x – 8x + 24
– 3x + 32 ≥ x + 24
x को बायीं ओर तथा 32 को दायीं ओर रखने पर
-3x – x ≥ 24 – 32
– 4x ≥ – 8
(-1) से गुणा करने पर तथा 4 से भाग देने पर।
x ≤ 84 या x ≤ 2
हल है: x ∈ (-∞, 2].

प्रश्न 17 से 20 तक की असमिकाओं को हल ज्ञात कीजिए तथा उन्हें संख्या रेखा पर आलेञ्चित कीजिए।

Ex 6.1 Class 11 Maths प्रश्न 17.
3x – 2 < 2x + 1
हल:
दी हुई असमिका . 3x – 2 < 2x + 1
2x को बायीं ओर तथा 2 को दायीं ओर रखने पर,
3x – 2x < 1 + 2
x < 3
हल है : x ∈ (-∞, 3).

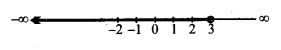
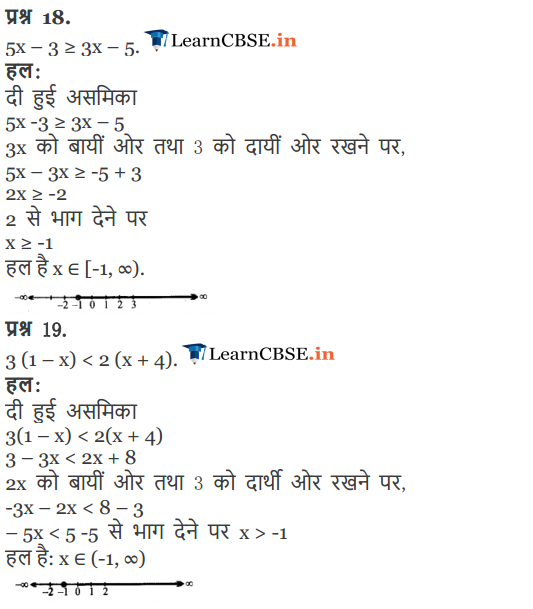
Ex 6.1 Class 11 Maths प्रश्न 18.
5x – 3 ≥ 3x – 5.
हल:
दी हुई असमिका
5x -3 ≥ 3x – 5
3x को बायीं ओर तथा 3 को दायीं ओर रखने पर,
5x – 3x ≥ -5 + 3
2x ≥ -2
2 से भाग देने पर
x ≥ -1
हल है x ∈ [-1, ∞).

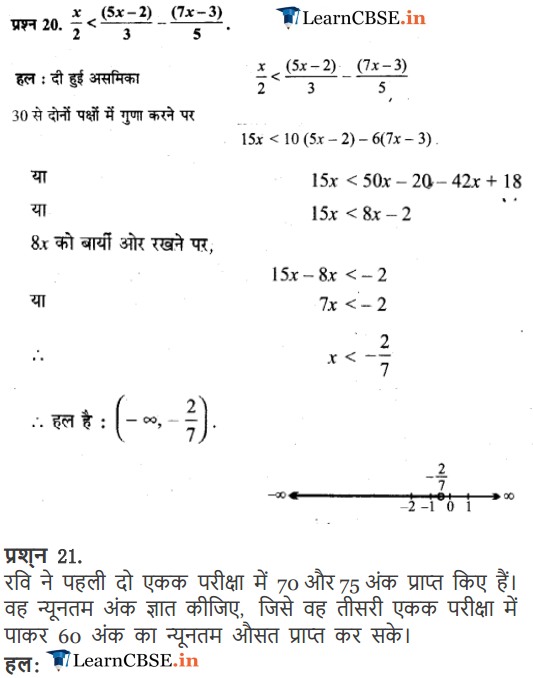
Ex 6.1 Class 11 Maths प्रश्न 19.
3 (1 – x) < 2 (x + 4).
हल:
दी हुई असमिका
3(1 – x) < 2(x + 4)
3 – 3x < 2x + 8
2x को बायीं ओर तथा 3 को दार्थी ओर रखने पर,
-3x – 2x < 8 – 3
– 5x < 5 -5 से भाग देने पर x > -1
हल है: x ∈ (-1, ∞)

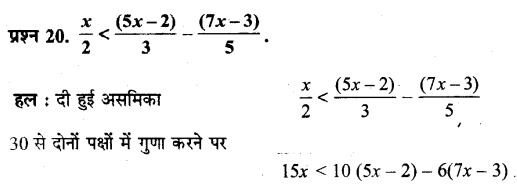
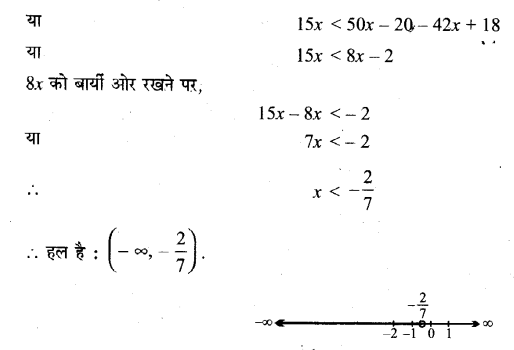
Ex 6.1 Class 11 Maths प्रश्न 21.
रवि ने पहली दो एकक परीक्षा में 70 और 75 अंक प्राप्त किए हैं। वह न्यूनतम अंक ज्ञात कीजिए, जिसे वह तीसरी एकक परीक्षा में पाकर 60 अंक का न्यूनतम औसत प्राप्त कर सके।
हल:
मान लीजिए तीसरे एकक परीक्षा में x अंक प्राप्त किए।

Ex 6.1 Class 11 Maths प्रश्न 22.
किसी पाठ्यक्रम में ग्रेड A पाने के लिए एक व्यक्ति को सभी पाँच परीक्षाओं (प्रत्येक 100 अंकों में से) में 90 अंक या अधिक अंक का औसत प्राप्त करना चाहिए यदि सुनीता के प्रथम चार परीक्षाओं के प्राप्तांक 87, 92, 94 और 95 हों तो वह न्यूनतम अंक ज्ञात कीजिए जिसे पांचवीं परीक्षा में प्राप्त करके सुनीता उस पाठ्यक्रम में ग्रेड A पाएगी।

Ex 6.1 Class 11 Maths प्रश्न 23.
10 से कम क्रमागत विषम संख्याओं के ऐसे युग्म ज्ञात कीजिए जिनके योगफल 11 से अधिक हों।
हल:
मान लीजिए x और x + 2 दो विषम परिमेय संख्याएँ हैं।
x तथा x + 2 दोनों ही 10 से कम हैं।
⇒ x < 10 और x + 2 < 10 या x < 8 दोनों का योगं 11 से अधिक है। x + (x + 2) > 11
2x + 2 > 11 या 2x > 11 – 2
2x > 9 या x > 92 या x > 412
अर्थात् यदि x = 5 हो, तब दूसरी संख्या = x + 2 = 7
इसी प्रकार यदि x = 7, तो x + 2 = 9
दूसरा युग्म (7, 9)
x = 9 नहीं हो सकता क्योंकि x + 2 = 11 > 10
अत: वांछित युग्म है (5, 7), 7, 9).

Ex 6.1 Class 11 Maths प्रश्न 24.
क्रमागत सर्म संख्याओं के ऐसे युग्म ज्ञात कीजिए जिनमें से प्रत्येक 5 से बड़े हों, तथा उनका योगफल 23 से कम हो।
हल:
मान लीजिए x और x + 2 दो सम संख्याएँ हैं।
x और x + 2 दोनों ही 5 से बड़ी है।
⇒ x > 5
x + (x + 2) < 23
2x + 2 < 23
2x < 23 – 2 = 21
2x < 21 या x < 212
यदि x = 10, x + 2 = 12 ⇒ x + (x + 2) < 23
इसी प्रकार (6, 8), (8, 10) युग्म भी दी हुई शर्त पूरी करते हैं। वांछित युग्म (6, 8), (8, 10), (10, 12).

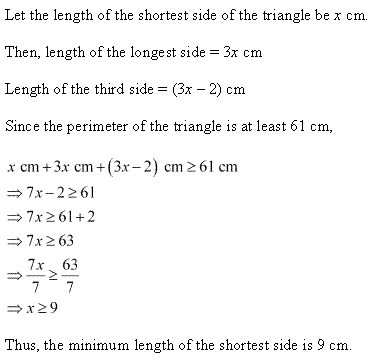
Ex 6.1 Class 11 Maths प्रश्न 25.
एक त्रिभुज की सबसे बड़ी भुजा सबसे छोटी भुजा की तीन गुनी है तथा त्रिभुज की तीसरी भुजा सबसे बड़ी भुजा से 2 सेमी कम है। तीसरी भुजा की न्यूनतम लंबाई ज्ञात कीजिए जबकि त्रिभुज का परिमाप न्यूनतम 61 सेमी है।
हल:
मान लीजिए त्रिभुज की सबसे छोटी भुजा = x सेमी
सबसे बड़ी भुजा = 3x सेमी
तीसरी भुजा = 3x – 2 सेमी
प्रश्नानुसार
x + 3x + (3x – 2) ≥ 61
7x – 2 ≥ 61
7x ≥ 61 + 2 = 63
x ≥ 9
सबसे छोटी भुजा 9 सेमी है।

Ex 6.1 Class 11 Maths प्रश्न 26.
एक व्यक्ति 91 सेमी लंबे बोर्ड में से तीन लंबाईयाँ काटना चाहता है। दूसरी लंबाई सबसे छोटी लंबाई से 3 सेमी अधिक और तीसरी लंबाई सबसे छोटी लंबाई की दूनी है। सबसे छोटे बोर्ड की संभावित लंबाई क्या है, यदि तीसरा टुकड़ा दूसरे टुकड़े से कम से कम 5 सेमी अधिक लंबा हो ?
हल:
मान लीजिए कटे हुए सबसे छोटे बोर्ड की लंबाई = x सेमी
दूसरे कटे हुए बोर्ड की लम्बाई = x + 3
तीसरे कटे हुए बोर्ड की लम्बाई = 2x सेमी
दिया है कि
x + (x + 3) + 2x ≤ 91
4x + 3 ≤ 91
4x ≤ 91 – 3 = 88
4x ≤ 88
x ≤ 22 ……(1)
यह भी दिया गया है कि 2x ≥ (x + 3) + 5
2x ≥ x + 8
x ≥ 8 ……(2)
सबसे छोटे बोर्ड की लम्बाई कम से कम 8 सेमी हो और अधिक से अधिक 22 सेमी हो।