NCERT Solutions For Class 6 Maths Chapter 14 Practical Geometry Ex 14.1

Exercise 14.1

Ex 14.1 Class 6 Maths Question 1.
Draw a circle of radius 3.2 cm.
Solution:
Step I : Mark a point O as a centre.
Step II: Open the compass up to the given radius 3.2 cm.

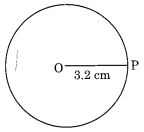
Step III : Put the needle of the compass at the centre O.
Step IV : Holding the top of the compass take one full round with pencil. The figure thus obtained is the required circle of radius 3.2 cm.

Ex 14.1 Class 6 Maths Question 2.
With the same centre O, Draw two circles of radius 4 cm and 2.5 cm.
Solution:
Step I : Take centre O and open the compass up to 4 cm.

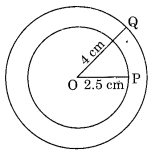
Step II : Draw a circle keeping the needle fixed at O.
Step III : Take the same centre O and open the compass up to 2.5 cm, and draw another circle.
The figure shows the required two circles with the same centre.

Ex 14.1 Class 6 Maths Question 3.
Draw a circle and any two its diameters. If you join the ends of these diameters, what is the figure obtained? What figure is obtained if the diameters are perpendicular to each other? How do you check your answer?
Solution:

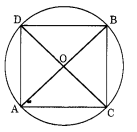
(i) Draw a circle with centre 0 with suitable radius.
(ii) AB and CD are any two diameters.
(iii) On joining the end points of the diameters, we get a quadrilateral ACBD.
(iv) We note that OA = OB = OC = OD [Same radius]
and AC = DB, AD = BC
∠A = ∠C = ∠B = ∠D = 90°
Thus ACBD is a rectangle.
Again if the diameters are perpendicular to each other then on measuring, we get
AC = DB = AD = BC
Thus, ACBD is a square.

Ex 14.1 Class 6 Maths Question 4.
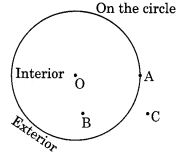
Draw any circle and mark points A, B and C such that
(a) A is on the circle
(b) B is in the interior of the circle
(c) C is in the exterior of the circle.
Solution:
Draw a circle with centre 0 and a suitable radius.
Here
(a) A is on the circle.
(b) B is in the interior of the circle.
(c) C is in the exterior of the circle.

Ex 14.1 Class 6 Maths Question 5.
Let A, B be the centres of the two circles of equal radii. Draw them so that each one of them passes through the centre of the other. Let them intersect at C and D.
Examine whether AB¯¯¯¯¯¯¯¯ and CD¯¯¯¯¯¯¯¯ are at right angles.
Solution:

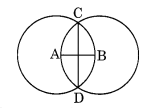
In the given figure two circles of equal radii intersect each other at C and D on measuring, we see that AB¯¯¯¯¯¯¯¯ and CD¯¯¯¯¯¯¯¯ intersect each other at right angles.

NCERT Solutions For Class 6 Maths Chapter 14 Practical Geometry Ex 14.2

Exercise 14.2

Ex 14.2 Class 6 Maths Question 1.
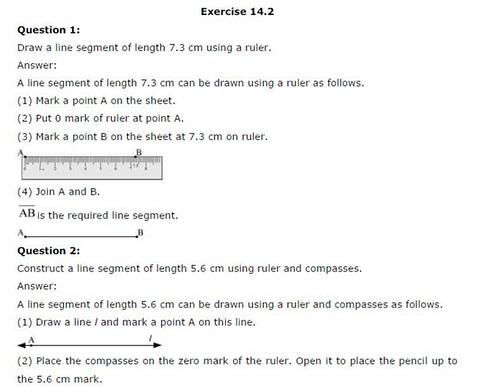
Draw a line segment of length 7.3 cm using ruler.
Solution:
Step I: Mark at point P.
Step II : Place the O mark of the ruler against the point P.
Step III : Mark a point Q at a distance of 7.3 cm from P.
Step IV : Join P and Q.

Thus PQ¯¯¯¯¯¯¯¯ is the line segment of length 7.3 cm.

Ex 14.2 Class 6 Maths Question 2.
Construct a line segment of length 5.6 cm using ruler and compass.
Solution:
Step I: Draw any line L of suitable lengths.
Step II : Place the needle of the compass on the zero mark of the ruler and open it upto 5.6 mark.
Step III : Place the needle at any point A at the line and draw an arc to cut l at B.

Thus, AB¯¯¯¯¯¯¯¯ is the required line segment of length 5.6 cm.

Ex 14.2 Class 6 Maths Question 3.
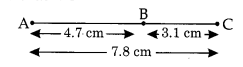
Construct AB¯¯¯¯¯¯¯¯ of length 7.8 cm. From this, cut off AC¯¯¯¯¯¯¯¯ of length 4.7 cm. Measure BC¯¯¯¯¯¯¯¯ .
Solution:
Given that AB¯¯¯¯¯¯¯¯ = 7.8 cm and AC¯¯¯¯¯¯¯¯ = 4.7 cm.
Step I : Place zero mark of the ruler at A.
Step II : Mark a point B at a distance of 7.8 cm from A.
Step III : Mark another point C at a distance of 4.7 cm from A such that AC = 4.7 cm.
Step IV : On measuring the length of BC, we find that BC¯¯¯¯¯¯¯¯ = 3.1 cm.

Ex 14.2 Class 6 Maths Question 4.
Given AB¯¯¯¯¯¯¯¯ of length 3.9 cm. Construct PQ¯¯¯¯¯¯¯¯ such that the length of PQ¯¯¯¯¯¯¯¯ is twice that of AB¯¯¯¯¯¯¯¯. Verify by measurement.

(Hint : Construct PX¯¯¯¯¯¯¯¯ such that the length of PX¯¯¯¯¯¯¯¯ = length of AB¯¯¯¯¯¯¯¯ then cut off XQ¯¯¯¯¯¯¯¯ such that XQ¯¯¯¯¯¯¯¯ also has the length of AB¯¯¯¯¯¯¯¯.
Solution:
Step I: Draw a line l of suitable length.
Step II: Draw AB¯¯¯¯¯¯¯¯ = 3.9 cm
Step III: From the line, construct PX¯¯¯¯¯¯¯¯ = AB¯¯¯¯¯¯¯¯ = 3.9 cm.
Step IV: Again construct XQ¯¯¯¯¯¯¯¯= AB¯¯¯¯¯¯¯¯ =3.9 cm
Verification: PX¯¯¯¯¯¯¯¯ + XQ¯¯¯¯¯¯¯¯ = AB¯¯¯¯¯¯¯¯ + AB¯¯¯¯¯¯¯¯

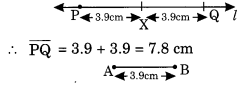
Thus twice of AB¯¯¯¯¯¯¯¯ is equal to PQ¯¯¯¯¯¯¯¯

Ex 14.2 Class 6 Maths Question 5.
Given AB¯¯¯¯¯¯¯¯ of length 7.3 cm and CD¯¯¯¯¯¯¯¯ of length 3.4 cm, construct a line segment XY¯¯¯¯¯¯¯¯ such that the length of XY is equal to the difference between the length of AB¯¯¯¯¯¯¯¯ and CD¯¯¯¯¯¯¯¯ . Verify the measurement.
Solution:
Step I : Construct AB¯¯¯¯¯¯¯¯ = 7.3 cm and CD¯¯¯¯¯¯¯¯ = 3.4 cm.

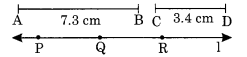
Step II: Take a point P on the given line l.
Step III: Construct PR¯¯¯¯¯¯¯¯ such that PR¯¯¯¯¯¯¯¯ = AB¯¯¯¯¯¯¯¯ = AB¯¯¯¯¯¯¯¯ = 7.3 cm.
Step IV:Construct RQ¯¯¯¯¯¯¯¯ = CD¯¯¯¯¯¯¯¯ = 3.4 cm such that PQ = AB¯¯¯¯¯¯¯¯ – CD¯¯¯¯¯¯¯¯ .
Verification : On measuring, we observe that PQ¯¯¯¯¯¯¯¯ = 3.9 cm = 7.3 cm 3.4 cm.
= AB¯¯¯¯¯¯¯¯ – CD¯¯¯¯¯¯¯¯
Thus, PQ¯¯¯¯¯¯¯¯ = AB¯¯¯¯¯¯¯¯ – CD¯¯¯¯¯¯¯¯.

NCERT Solutions For Class 6 Maths Chapter 14 Practical Geometry Ex 14.3

Exercise 14.3

Ex 14.3 Class 6 Maths Question 1.
Draw any line segment PQ¯¯¯¯¯¯¯¯ . Without measuring PQ¯¯¯¯¯¯¯¯, construct a copy of PQ¯¯¯¯¯¯¯¯.
Solution:
Step I: Draw PQ¯¯¯¯¯¯¯¯ of unknown length.
Step II : Draw a line l and mark a point A on it.
Step III: Open the compass equal to PQ.
Step IV : Place the needle of the compass at A and mark a point B on l.
Thus, AB¯¯¯¯¯¯¯¯ is a copy of PQ¯¯¯¯¯¯¯¯.

Ex 14.3 Class 6 Maths Question 2.
Given some line segment AB¯¯¯¯¯¯¯¯ whose length you do not know, construct PQ¯¯¯¯¯¯¯¯ such that the length of PQ¯¯¯¯¯¯¯¯ is twice that of AB¯¯¯¯¯¯¯¯.
Solution:
Step I : Draw AB¯¯¯¯¯¯¯¯ of any suitable length.
Step II : Place the needle of the compass at A and the other pencil end at B.
Step III : Draw a line l and take a point P on it.
Step IV: With the same opening of the compass, place the needle at P and mark another point Q on l.
Thus PQ¯¯¯¯¯¯¯¯ is the required line segment whose length is twice the length of AB¯¯¯¯¯¯¯¯ i.e PQ¯¯¯¯¯¯¯¯ = 2AB¯¯¯¯¯¯¯¯¯¯ .

NCERT Solutions For Class 6 Maths Chapter 14 Practical Geometry Ex 14.4

Exercise 14.4

Ex 14.4 Class 6 Maths Question 1.
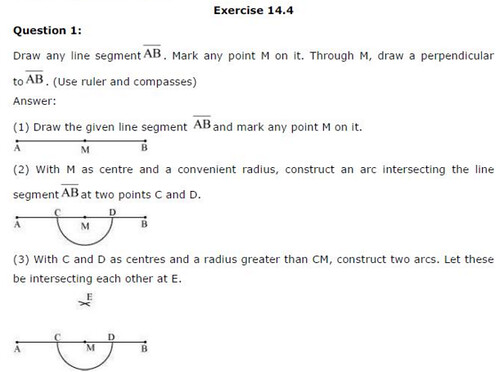
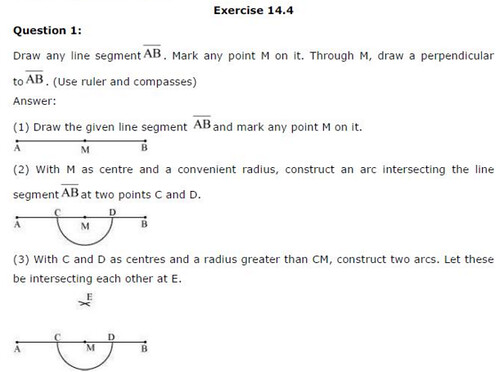
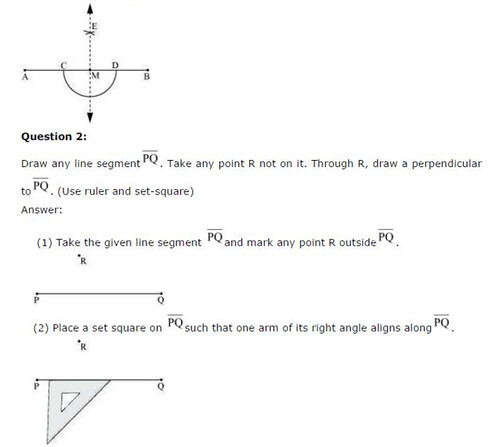
Draw any line segment AB¯¯¯¯¯¯¯¯. Make any point M on it. Through M, draw a perpendicular to AB¯¯¯¯¯¯¯¯. (Use ruler and Compasses)
Solution:
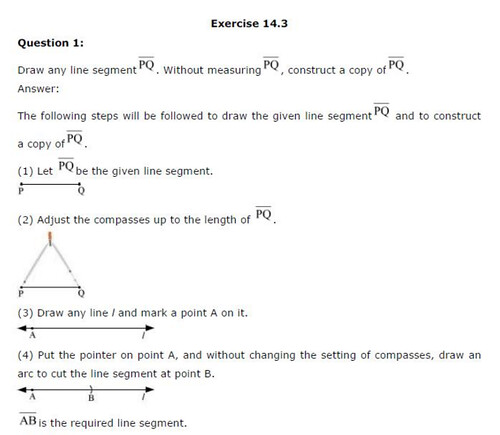
Step I : Draw a line segment AB¯¯¯¯¯¯¯¯ and mark any point M on it.
Step II : Put the pointer of the compass at M and draw an arc of suitable radius such that it intersects AB¯¯¯¯¯¯¯¯ at P and Q.

Step III : Take P and Q as centres and radius greater than PM, draw two arcs such that they intersect each other at C.
Step IV : Join M and C.
Thus CM is the perpendicular to AB¯¯¯¯¯¯¯¯.

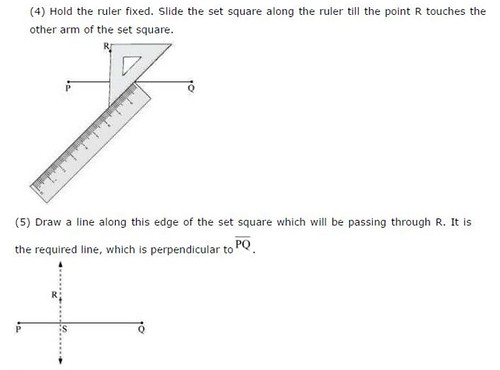
Ex 14.4 Class 6 Maths Question 2.
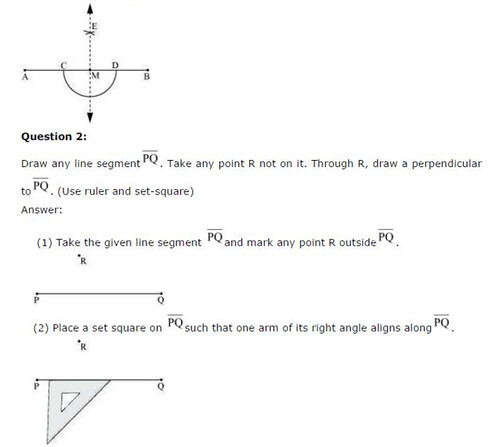
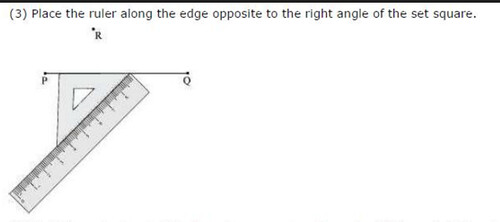
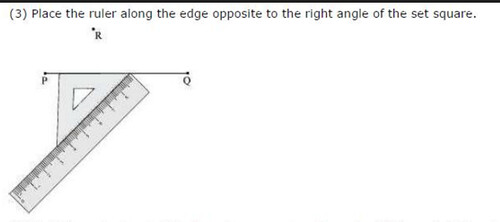
Draw any line segment PQ¯¯¯¯¯¯¯¯. Take any point R not on it. Through R, draw a perpendicular to PQ¯¯¯¯¯¯¯¯. (Use ruler and set square).
Solution:
Step I: Draw a line segment PQ¯¯¯¯¯¯¯¯ and a point R outside of PQ¯¯¯¯¯¯¯¯.
Step II : Place a set square on PQ¯¯¯¯¯¯¯¯ such that one side of its right angle be along it.
Step III: Place a ruler along the longer side of the set square.

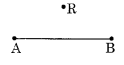
Step IV : Hold the ruler fix and slide the set square along the ruler till it touches the point R.

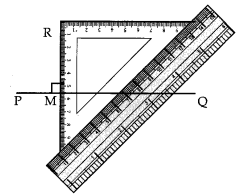
Step V : Join RM along the edge through R. Thus RM¯¯¯¯¯¯¯¯¯ ⊥ PQ¯¯¯¯¯¯¯¯.

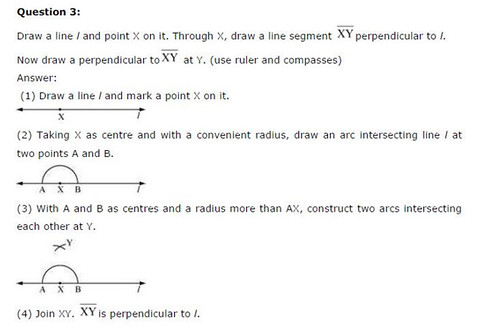
Ex 14.4 Class 6 Maths Question 3.
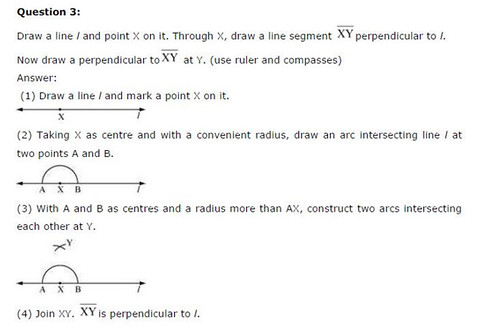
Draw a line l and a point X on it. Through X, draw a line segment XY¯¯¯¯¯¯¯¯ perpendicular to l. Now draw a perpendicular to XY¯¯¯¯¯¯¯¯ at y. (Use ruler and compasses)
Solution:
Step I: Draw a line l and take a point X on it.
Step II : Draw an arc with centre X and of suitable radius to intersect the line l at two points P and Q.

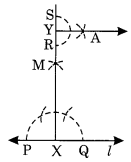
Step III : With P and Q as centres and a radius greater than P draw two arcs to intersect each other at M.
Step IV : Join XM and produce to Y.
Step V : With Y as centre and a suitable radius, draw an arc to intersect XY at two points R and S.
Step VI: With R and S as centres and a radius greater than YR, draw two arcs to intersect each other at A.
Step VII: Join Y and A. Thus YA ⊥ XY.

NCERT Solutions For Class 6 Maths Chapter 14 Practical Geometry Ex 14.5

Exercise 14.5

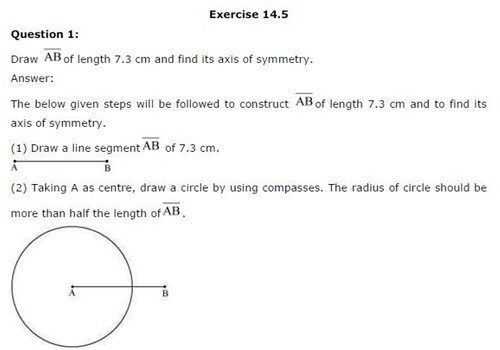
Ex 14.5 Class 6 Maths Question 1.
Draw AB of length 7.3 cm and find its axis of symmetry.
Solution:
Step I: Draw AB¯¯¯¯¯¯¯¯ = 7.3 cm

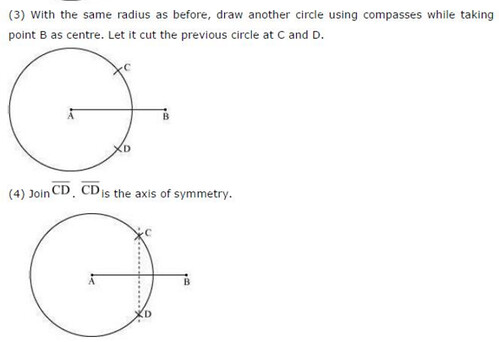
Step II: Taking A and B as centre and radius more than half of AB¯¯¯¯¯¯¯¯, draw two arcs which intersect each other at C and D.
Step III: Join C and D to intersect AB¯¯¯¯¯¯¯¯ at E. Thus, CD is the perpendicular bisector or axis of symmetry of AB¯¯¯¯¯¯¯¯.

Ex 14.5 Class 6 Maths Question 2.
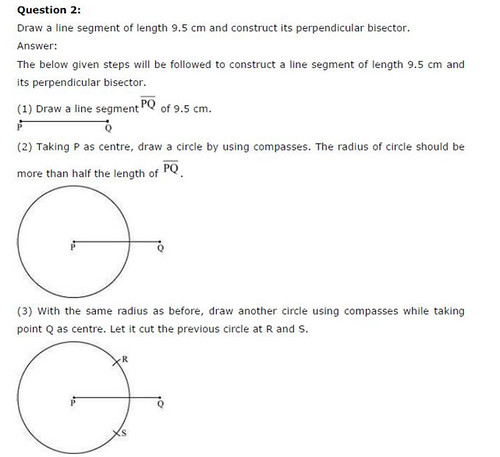
Draw a line segment of length 9.5 cm and construct its perpendicular bisector.
Solution:
Step I: Draw a line segment PQ¯¯¯¯¯¯¯¯ =9.5 cm

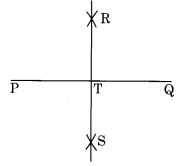
Step II: With centres P and Q and radius more than half of PQ, draw two arcs which meet each other at R and S.
Step III: Join R and S to meet PQ¯¯¯¯¯¯¯¯ at T.
Thus, RS is the perpendicular bisector of PQ.

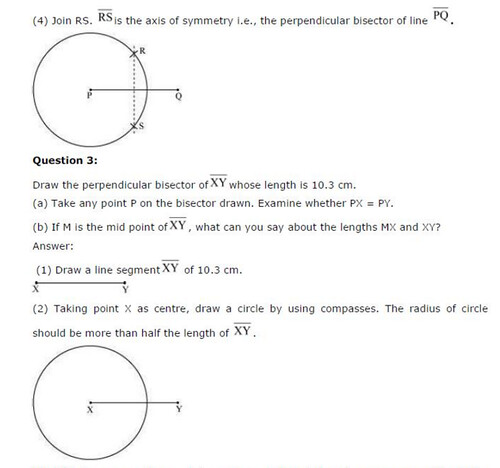
Ex 14.5 Class 6 Maths Question 3.
Draw the perpendicular bisector of XY¯¯¯¯¯¯¯¯ whose length is 10.3 cm.
(a) Take any point P on the bisector drawn. Examine whether PX = PY.
(b) If M is the midpoint of XY¯¯¯¯¯¯¯¯ . What can you say about the length of MX and MY?
Solution:
Step I: Draw a line segment XY¯¯¯¯¯¯¯¯ = 10.3 cm.

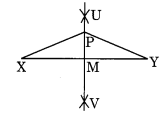
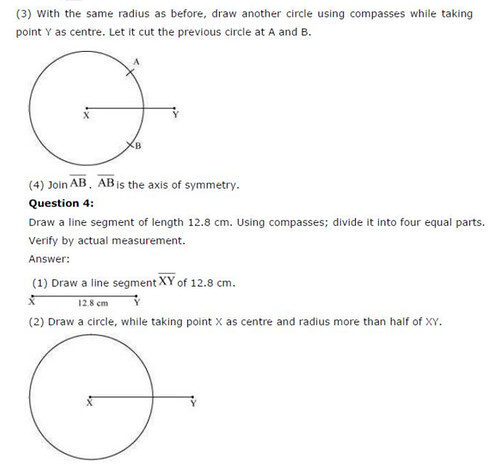
Step II : With centre X and Y and radius more than half of XY, draw two arcs which meet each other at U and V.
Step III: Join U and V which meets XY¯¯¯¯¯¯¯¯ at M.
Step IV: Take a point P on UV¯¯¯¯¯¯¯¯ .
(a) On measuring, PX = PY = 5.6 cm.
(b) On measuring, MX¯¯¯¯¯¯¯¯¯¯ = MY¯¯¯¯¯¯¯¯¯ = 12 XY = 5.15 cm.

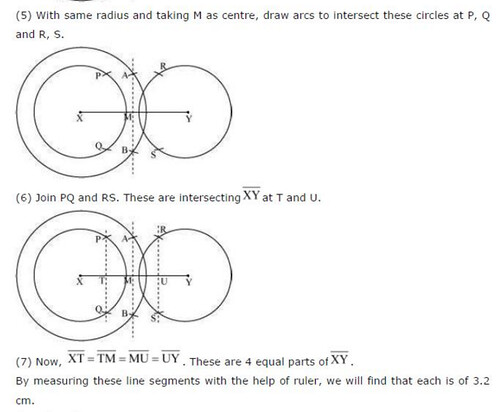
Ex 14.5 Class 6 Maths Question 4.
Draw a line segment of length 12.8 cm. Using compasses, divide it into four equal parts. Verify by actual measurement.
Solution:
Step I: Draw a line segment AB¯¯¯¯¯¯¯¯ = 12.8 cm

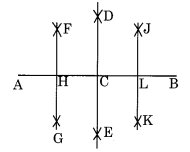
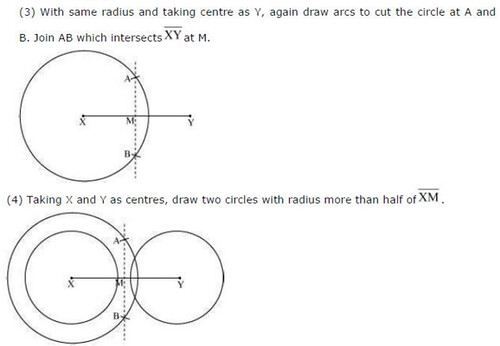
Step II : With centre A and B and radius more than half of AB, draw two arcs which meet each other at D and E.
Step III : Join D and E which meets AB¯¯¯¯¯¯¯¯ at C which is the midpoint of AB¯¯¯¯¯¯¯¯.
Step IV : With centre A and C and radius more than half of AC, draw two arcs which meet each other at F and G.
Step V: Join F and G which meets AC¯¯¯¯¯¯¯¯ at H which is the midpoint of AC¯¯¯¯¯¯¯¯ .
Step VI : With centre C and B and radius more than half of CB, draw two arcs which meet each other at J and K.
Step VII : Join J and K which meets CB¯¯¯¯¯¯¯¯ at L which is the midpoint of CB¯¯¯¯¯¯¯¯ .
Thus, on measuring, we find
AH¯¯¯¯¯¯¯¯ = HC¯¯¯¯¯¯¯¯ = CL¯¯¯¯¯¯¯ = LB¯¯¯¯¯¯¯ = 3.2 cm.

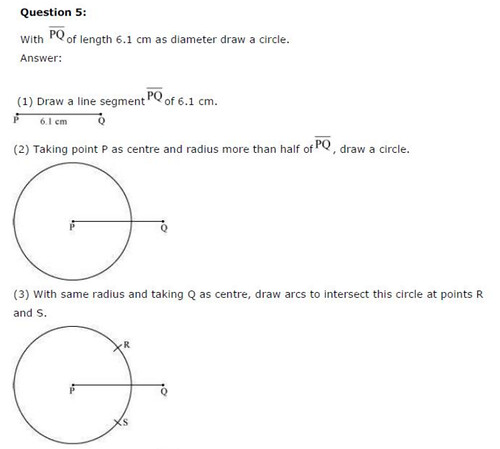
Ex 14.5 Class 6 Maths Question 5.
With PQ¯¯¯¯¯¯¯¯ of length 6.1 cm as diameter, draw a circle.
Solution:
Step I: Draw PQ¯¯¯¯¯¯¯¯ = 6.1 cm
Step II: Draw a perpendicular bisector of PQ¯¯¯¯¯¯¯¯ which meets PQ¯¯¯¯¯¯¯¯ at R i.e. R is the midpoint of PQ¯¯¯¯¯¯¯¯.

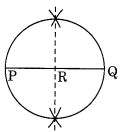
Step III : With centre R and radius equal to RP¯¯¯¯¯¯¯¯ , draw a circle passing through P and Q.
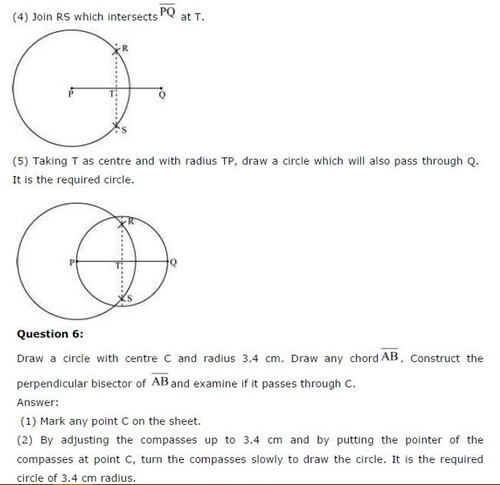
Thus, the circle with diameter PQ¯¯¯¯¯¯¯¯ = 6.1 cm is the required circle.

Ex 14.5 Class 6 Maths Question 6.
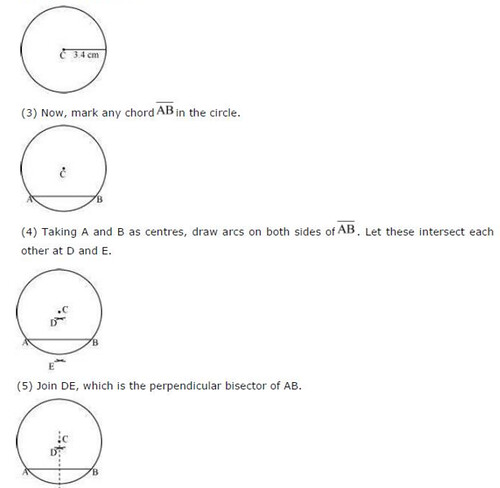
Draw a circle with centre C and radius 3.4 cm. Draw any chord AB¯¯¯¯¯¯¯¯ . Construct the perpendicular bisector of AB¯¯¯¯¯¯¯¯ and examine if it passes through C.
Solution:
Step I: Draw a circle with centre C and radius 3.4 cm.
Step II: Draw any chord AB¯¯¯¯¯¯¯¯.
Step III : Draw the perpendicular bisector of AB¯¯¯¯¯¯¯¯ which passes through the centre C.

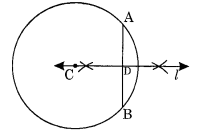
Ex 14.5 Class 6 Maths Question 7.
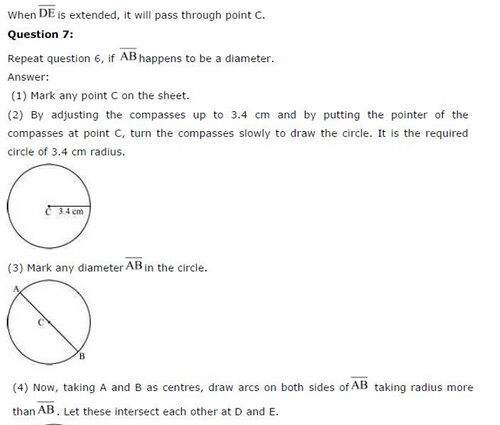
Repeat Question number 6, if AB¯¯¯¯¯¯¯¯ happens to be a diameter.
Solution:
Step I: Draw a circle with centre C and radius 3.4 cm.
Step II : Draw a diameter AB of the circle.

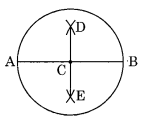
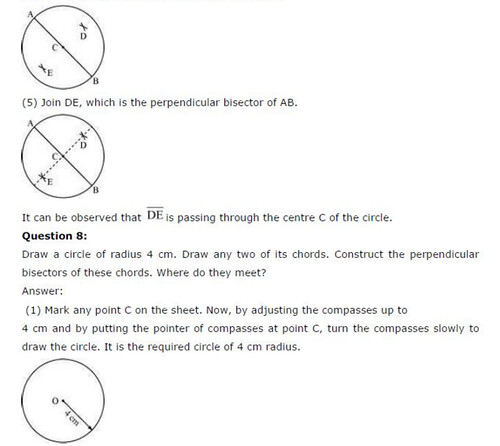
Step III : Draw a perpendicular bisector of AB which passes through the centre C and on measuring, we find that C is the midpoint of AB¯¯¯¯¯¯¯¯ .

Ex 14.5 Class 6 Maths Question 8.
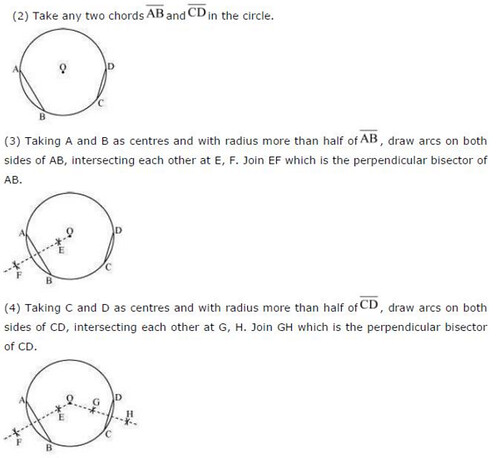
Draw a circle of radius 4 cm. Draw any two of its chords. Construct the perpendicular bisectors of these chords. Where do they meet?
Solution:
Step I: Draw a circle with centre 0 and radius 4 cm.

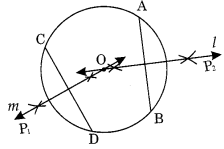
Step II: Draw any two chords AB¯¯¯¯¯¯¯¯ and CD¯¯¯¯¯¯¯¯ of the circle.
Step III : Draw the perpendicular bisectors of AB¯¯¯¯¯¯¯¯ and CD¯¯¯¯¯¯¯¯ i.e. I and m.
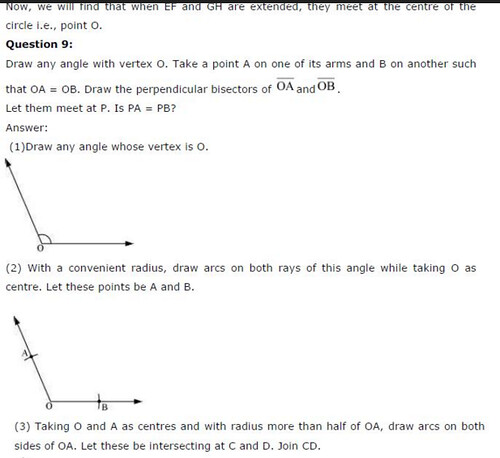
Step IV : On producing the two perpendicular bisectors meet each other at the centre O of the circle.

Ex 14.5 Class 6 Maths Question 9.
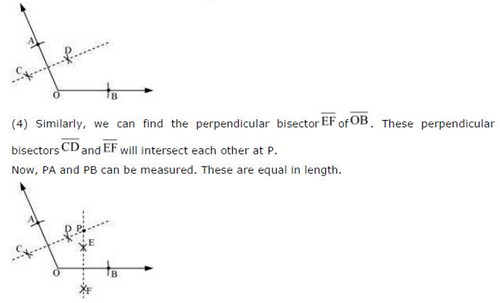
Draw any angle with vertex O. Take a point A on one of its arms and B on another such that OA = OB. Draw the perpendicular bisectors of OA¯¯¯¯¯¯¯¯ and OB¯¯¯¯¯¯¯¯ . Let them meet at P. Is PA = PB?
Solution:
Step I: Draw an angle XOY with O as its vertex.
Step II : Take any point A on OY and B on OX, such that OA + OB.

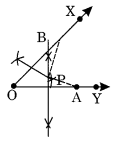
Step III : Draw the perpendicular bisectors of OA and OB which meet each other at a point P.
Step IV : Measure the lengths of PA¯¯¯¯¯¯¯¯ and PB¯¯¯¯¯¯¯¯. Yes, PA¯¯¯¯¯¯¯¯ = PB¯¯¯¯¯¯¯¯.

NCERT Solutions For Class 6 Maths Chapter 14 Practical Geometry Ex 14.6

Exercise 14.6

Ex 14.6 Class 6 Maths Question 1.
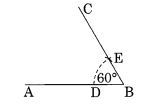
Draw ∠POQ of measure 75° and find its line of symmetry.
Solution:
Step I : Draw a line segment PQ¯¯¯¯¯¯¯¯ .
Step II : With centre Q and suitable radius, draw an arc to cut PQ at R.

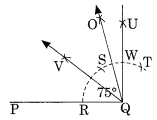
Step III : With centre R and radius of the same length, mark S and T on the former arc.
Step IV : With centres S and T and with the same radius, draw two arcs which meet each other at U.
Step V: Join QU such that ∠PQU = 90°.
Step VI : With centres S and W, draw two arcs of the same radius which meet each other at Q.
Step VII: Join Q and O such that ∠PQO = 75°.
Step VIII: Bisect ∠PQO with QV.
Thus, OV is the line of symmetry of ∠PQO.

Ex 14.6 Class 6 Maths Question 2.
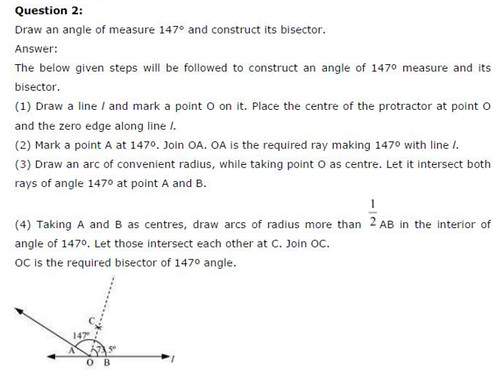
Draw an angle of measure 147° and construct its bisector.
Solution:
Step I : Draw ∠ABC = 147° with the help of protractor.

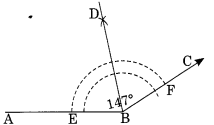
Step II : With centres B and radius of proper length, draw an arc which meets AB and AC at E and F respectively.
Step III : With centres E and F and the radius more that half of the length of arc EF, draw two arcs which meet each other at D.
Step IV : Join B and D.
Thus, BD is the bisector of ∠ABC.

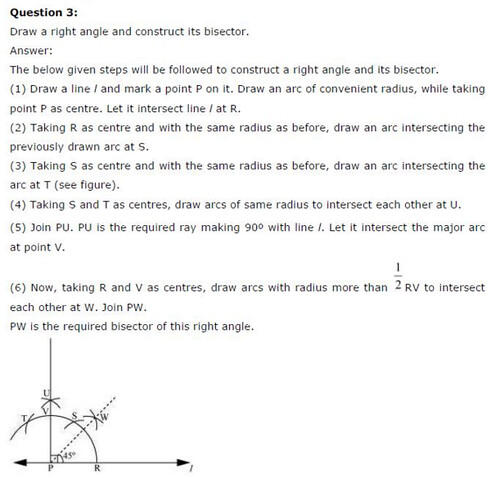
Ex 14.6 Class 6 Maths Question 3.
Draw a right angle and construct its bisector.
Solution:
Step I: Draw a line segment AB.
Step II : With centre B and proper radius draw an arc to meet AB at C.

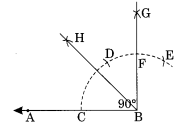
Step III : With centre C and same radius, mark two marks D and E on the former arc.
Step IV : With centres D and E and the same radius, draw two arcs which meet each other at G.
Step V : Join B and G such that ∠ABG = 90°
Step VI : Draw BH as the bisector of ∠ABG such that ∠ABH = 45°.
Thus ∠ABG is the right angle and BH is the bisector of ∠ABG.

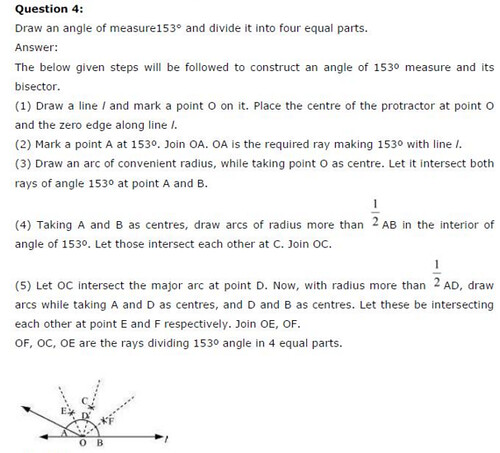
Ex 14.6 Class 6 Maths Question 4.
Draw an angle of 153° and divide it into four equal parts.
Solution:
Step I : Draw ∠ABP = 153° with the help of protractor.

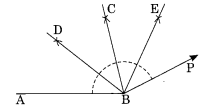
Step II : Draw BC as the bisector of ∠ABP which dividers ∠ABP into two equal parts.
Step III : Draw BD and BE as the bisector of ∠ABC and ∠CBP respectively.
Thus, the bisectors BD, BC and BE divide the ∠ABP into four equal parts.

Ex 14.6 Class 6 Maths Question 5.
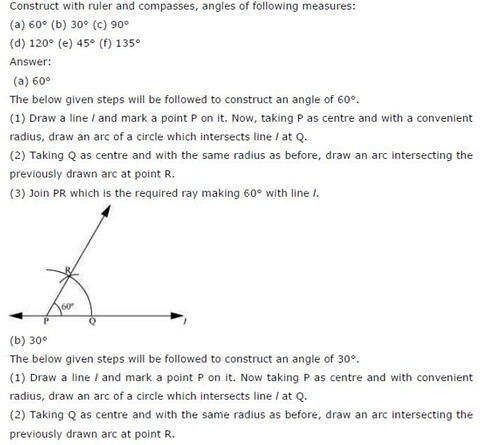
Construct with ruler and compasses, angles of the following measures:
(a) 60°
(b) 30°
(c) 90°
(d) 120°
(e) 45°
(f) 135°
Solution:
(a) Angle of 60°

Step I: Draw a line segment AB¯¯¯¯¯¯¯¯ .
Step II : With centre B and proper radius draw an arc.
Step III : With centre D and radius of the- same length mark a point E on the former arc.
Step IV : Join B to E and product to C. Thus ∠ABC is the required angle of measure 60°.

(b) Step I: Draw ∠ABC = 60° as we have done in section (a).
Step II: Draw BF as the bisector of ∠ABC.

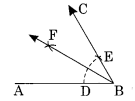
Thus ∠ABF = 602 = 30°.

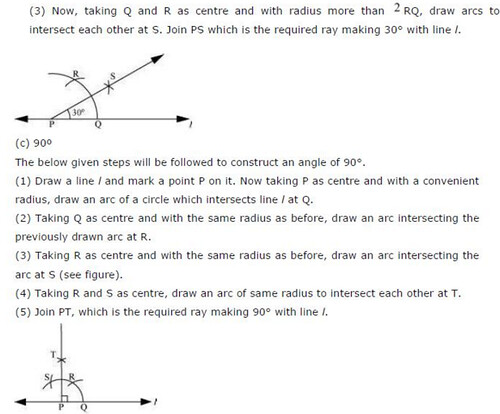
(c) Angle of 90°
In the given figure,
∠ABC = 90°(Refer to solution 3)

(d) Angle of 120°.
Step I: Draw AB¯¯¯¯¯¯¯¯
Step II : With centre A and radius of proper length, draw an arc.

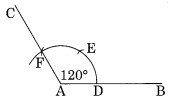
Step III : With centre D and the same radius, draw two mark E and F on former arc.
Step IV : Join A to F and produce to C. Thus ∠CAB = 120°

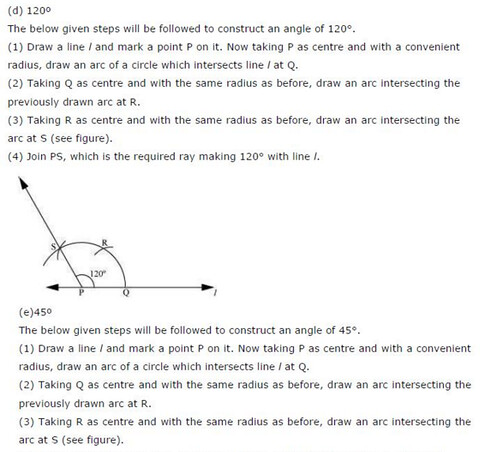
(e) Angle of 45s, i.e., 902 = 45°
In the figure ∠ABD = 45° (Refer to solution 3)

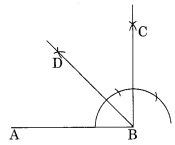
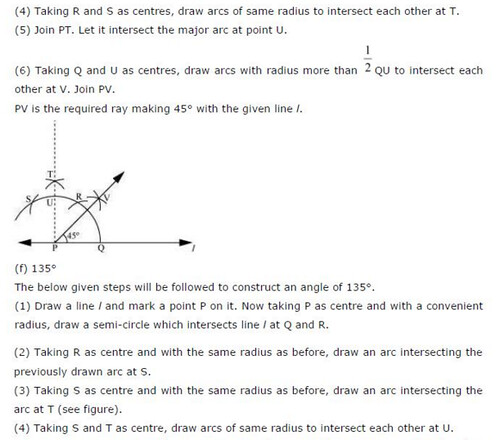
(f) An angle of 135°
Since 135° = 90° + 45°
= 90° + (902 )°
In this figure ∠ABC = 135°

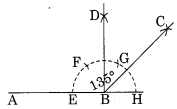
Ex 14.6 Class 6 Maths Question 6.
Draw an angle of measure 45° and bisect it.
Solution:
Step I : Draw a line AB and take any point O on it.
Step II: Construct ∠AOE = 45° at O.

Step III: With centre O and proper radius, draw an arc GF.
Step IV : With centres G and F and proper radius, draw two arcs which intersect each other at D.
Step V : Join O to D.
Thus ∠AOE = 45° and OD is its bisector.

Ex 14.6 Class 6 Maths Question 7.
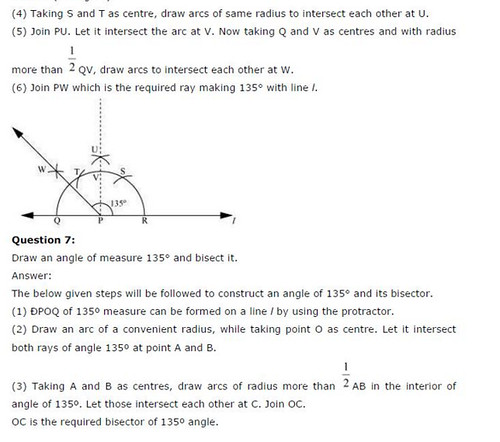
Draw an angle of measure 135° and bisect it.
Solution:
Steps I: Draw a line OA and take any point P on it.

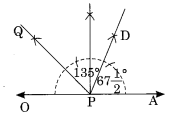
Step II: Construct ∠APQ = 135°.
Step III : Draw PD as the bisector of angle APQ.
Thus ∠APQ = 13502 = 67 102.

Ex 14.6 Class 6 Maths Question 8.
Draw an angle of 70°. Make a copy of it using only a straight edge and compasses.
Solution:
Step I : Draw a line AB and take any point 0 on it.
Step II : Draw ∠COB = 70° using protractor.

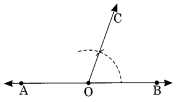
Step III: Draw a ray PQ−→− .
Step IV: With centre O and proper radius, draw an arc which meets OA−→− and OB−→− at E and F respectively.

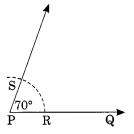
Step V : With the same radius and centre at P, draw an arc meeting PQ−→− at R.
Step VI: With centre R and keeping and radius equal to EF, draw an arc intersecting the former arc at S.
Step VII : Join P and S and produce it. Thus, QPS is the copy of AOB = 70°.

Ex 14.6 Class 6 Maths Question 9.
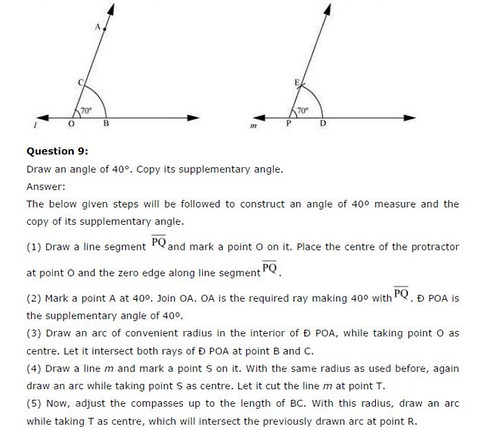
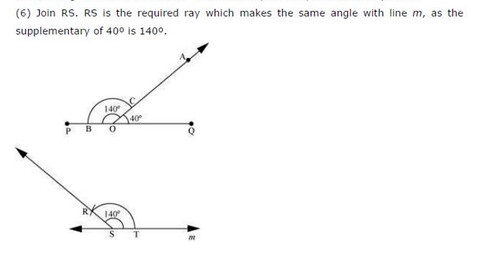
Draw an angle of 40°. Copy its supplementary angle.
Solution:
Step I: Construct ∠AOB = 40° using protractor.
∠COF is the supplementary angle of ∠AOB.

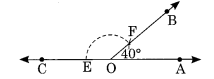
Step II : Draw a ray PR−→− and take any point Q on it.
Step III : With centre O and proper radius, draw an arc which intersects OC−→− and OB−→− at E and F respectively.

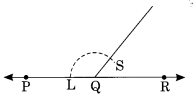
Step IV : With centre Q and same radius, draw an arc which intersects PQ−→− at L.
Step V: With centre L and radius equal to EF, draw an arc which intersects the former arc at S.
Step VI : Join Q and S and produce.
Thus, ∠PQS is the copy of the supplementary angle COB.